
|
|

|
Greg Egan and I have been thinking about Lorentzian lattices, and he just proved something nice about \(\mathrm{E}_{10}\): it's the lattice of \(2 \times 2\) self-adjoint matrices with integral octonions as entries!
This might be known already, but I've never seen it anywhere. So, let me show you the proof. But first, let me remind you why it matters.
\(\mathrm{E}_8\) is the name of four related things:
These things are endlessly absorbing, since they have deep connections to the icosahedron, the topology of 4-manifolds, string theory, and more. \(\mathrm{E}_8\) seems to be a wormhole in the fabric of mathematics, a portal that connects the most beautiful, special features of otherwise distant subjects.
For example: since the \(\mathrm{E}_8\) lattice lives in 8 dimensions, you can think of the vectors in this lattice as octonions! And if you rescale and rotate it correctly, this lattice becomes closed under octonion multiplication, as well as addition and subtraction. Then we call it the lattice of Cayley integers, or integral octonions:
$$\mathbf{O} \subseteq \mathbb{O}$$\(\mathbb{O}\) is the octonions, and \(\mathbf{O}\) is the integral octonions.
I explained exactly how this works last time, in Part 6.
\(\mathrm{E}_{8}\) has a big brother called \(\mathrm{E}_{10}\). Again this is the name of four things:
It's been known for a while that the symmetry group of the \(\mathrm{E}_{10}\) lattice has a nice description in terms of integral octonions:
Namely, the even part of this group, the transformations with determinant 1 rather than -1, is \(\mathrm{PSL}(2,\mathbf{O})\). Making sense of this group takes bit of work, since the octonions are nonassociative! But the paper above does it.
Recently Greg Egan and I have been thinking about this stuff, and a couple days ago he found something I'd long been hoping for: a description of the \(\mathrm{E}_{10}\) lattice itself in terms of integral octonions! It consists of \(2 \times 2\) self-adjoint matrices with entries in \(\mathbf{O}\).
This result is a discrete 10-dimensional analogue of a well-known 4-dimensional fact. Good old 4d Minkowksi spacetime can be seen as consisting of \(2 \times 2\) self-adjoint matrices with complex entries. Such matrices look like this:
$$ T = \left( \begin{array}{cc} t + z & x - i y \\ x + i y & t - z \end{array} \right) $$with \((t,x,y,z) \in \mathbb{R}^4\), and
$$ -\det (T) = x^2 + y^2 + z^2 - t^2 $$is the Minkowski metric. The group \(\mathrm{SL}(2,\mathbb{C})\) acts on these \(2 \times 2\) self-adjoint matrices via
$$ T \mapsto g T g^* $$and this action preserves the determinant. So, we get a map from \(\mathrm{SL}(2,\mathbb{C})\) to the Lorentz group! The kernel of this is \(\pm 1\), so the group
$$ \mathrm{PSL}(2,\mathbb{C}) = \mathrm{SL}(2,\mathbb{C})/ \{\pm I \} $$is a subgroup of the Lorentz group, and in fact it's the connected component of the identity:
$$ \mathrm{PSL}(2,\mathbb{C}) \cong \mathrm{SO}_0(3,1) $$The same sort of thing works for all four normed division algebras, though it gets kind of funky in the noncommutative and nonassociative cases:
$$ \begin{array}{ccl} \mathrm{PSL}(2,\mathbb{R})& \cong &\mathrm{SO}_0(2,1) \\ \mathrm{PSL}(2,\mathbb{C})& \cong &\mathrm{SO}_0(3,1) \\ \mathrm{PSL}(2,\mathbb{H})& \cong &\mathrm{SO}_0(5,1) \\ \mathrm{PSL}(2,\mathbb{O})& \cong &\mathrm{SO}_0(9,1) . \end{array} $$The same idea should explain why \(\mathrm{PSL}(2,\mathbf{O})\) is the even part of the symmetry group of the \(\mathrm{E}_{10}\) lattice. But implementing this demands that we see the \(\mathrm{E}_{10}\) lattice as consisting of \(2 \times 2\) matrices with entries in \(\mathbf{O}\), the integral octonions.
We already know that 10-dimensional Minkowski spacetime is isometric to the space of \(2 \times 2\) self-adjoint octonionic matrices:
$$ \mathfrak{h}_2(\mathbb{O}) = \left\{ \left( \begin{array}{cc} a & b \\ b^* & c \end{array} \right) \; : \; a,b,c \in \mathbb{O} , \; a = a^*, c=c^* \right\} $$with its 'Minkowski metric'
$$ - \det \left( \begin{array}{cc} a & b \\ b^* & c \end{array} \right) = |b|^2 - a c \in \mathbb{R} \subseteq \mathbb{O} $$(You see, an octonion that's its own conjugate can be identified with a real number.)
So, we want:
Theorem. Up to a rescaling, the \(\mathrm{E}_{10}\) lattice in 10-dimensional Minkowski spacetime is isometric to the set of \(2 \times 2\) self-adjoint matrices with integral octonions as entries: $$ \mathfrak{h}_2(\mathbf{O}) = \left\{ \; \left( \begin{array}{cc} a & b \\ b^* & c \end{array} \right) \; : \; a,b,c \in \mathbf{O} , \; a = a^*, c=c^* \; \right\} $$ viewed as a sublattice of \(\mathfrak{h}_2(\mathbb{O})\) with its Minkowski metric.
Proof. Up to isometry, \(\mathrm{E}_{10}\) is the unique lattice in 10-dimensional Minkowski spacetime with a basis described by this Dynkin diagram:
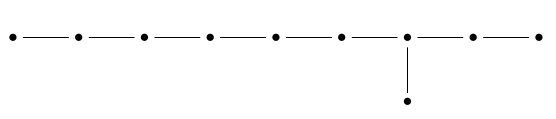
meaning that the basis vectors \(e_i\) (called 'simple roots') have inner products
$$ e_i \cdot e_i = 2 $$and for \(i \ne j\),
$$ e_i \cdot e_j = -1 $$when the corresponding dots in the diagram are connected by an edge, but
$$ e_i \cdot e_j = 0 $$when they're not. So, it suffices to find basis vectors for the lattice \(\mathfrak{h}_2(\mathbf{O})\) obeying identical relations up to rescaling, where the inner product is now the one coming from the Minkowski metric on \(\mathfrak{h}_2(\mathbb{O})\).
Since the shortest nonzero vectors in \(\mathfrak{h}_2(\mathbf{O})\) have length 1, we need to rescale by a factor of \(1/\sqrt{2}\). We thus seek 10 basis vectors \(v_i\) for the lattice \(\mathfrak{h}_2(\mathbf{O})\) obeying
$$ v_i \cdot v_i = 1 $$and for \(i \ne j\),
$$ v_i \cdot v_j = -\frac{1}{2} $$when the corresponding dots in the \(\mathrm{E}_{10}\) Dynkin diagram are connected by an edge, but
$$ v_i \cdot v_j = 0 $$otherwise.
Now, just as an octonion that's its own conjugate can be identified with a real number, an integral octonion that is its own conjugate can be identified with an integer. So, we have
$$ \mathfrak{h}_2(\mathbb{O}) = \left\{ \; \left( \begin{array}{cc} t + z & v \\ v^* & t-z \end{array} \right) \; : \; v \in \mathbb{O} , \; t, z \in \mathbb{R} \; \right\} $$and
$$ \mathfrak{h}_2(\mathbf{O}) = \left\{ \; \left( \begin{array}{cc} t + z & v \\ v^* & t-z \end{array} \right) \; : \; v \in \mathbf{O} , \; t + z, t - z \in \mathbb{Z} \; \right\} $$and in this description, the Minkowski metric is given by
$$ -t^2 + z^2 + |v|^2 $$Note that setting \(t\) and \(z\) to zero gives a way to identify any octonion \(v\) with an element of \(\mathfrak{h}_2(\mathbb{O})\), namely
$$ \left( \begin{array}{cc} 0 & v \\ v^* & 0 \end{array} \right) $$This lets us identify any octonionic integer with an element of \(\mathfrak{h}_2(\mathbf{O})\). The octonionic integers form a rescaled copy of the \(\mathrm{E}_8\) lattice. Since the Dynkin diagram for \(\mathrm{E}_8\):
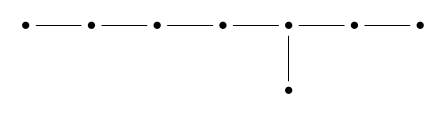
is contained in that for \(\mathrm{E}_{10}\), a good first step is to find 8 choices for \(v \in \mathbf{O}\) that correspond to rescaled versions of the simple roots for \(\mathrm{E}_8\).
To do this, we identify \(\mathbb{O}\) with \(\mathbb{R}^8\) in such a way that the first component is real and the other seven components are imaginary, and the multiplication table is the one I described last time using this mnemonic:
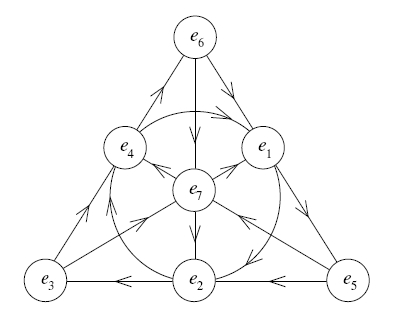
Given all this, Egan chose these octonions as his rescaled versions of the simple roots for \(\mathrm{E}_8\):
$$ \begin{array} {cccccccccc} v_1 &=& (-\frac{1}{2},& \frac{1}{2}, &-\frac{1}{2},& 0,& -\frac{1}{2},& 0,& 0,& 0) \\ v_2&=&(0,&0,&0,& 0,& 1,& 0,& 0,& 0) \\ v_3&=&(0, &0,& \frac{1}{2},& -\frac{1}{2}, &-\frac{1}{2},& 0,& 0, &-\frac{1}{2}) \\ v_4&=&(0, &0,& 0,& 0, &0, &0, &0,& 1) \\ v_5&=&(0, &0, &0, &\frac{1}{2},& 0,& -\frac{1}{2},& -\frac{1}{2},& -\frac{1}{2}) \\ v_6&=&(0,& 0,& 0,& 0,& 0, &0, &1, &0) \\ v_7&=&(\frac{1}{2},& 0,& -\frac{1}{2},& -\frac{1}{2},& 0,& 0,& -\frac{1}{2},& 0) \\ v_8&=&(0,& 0,& 0, &0, &0, &1,& 0, & 0) \end{array} $$The diligent reader will check that each of these arises by taking a Kirmse integer (a concept explained last time) and swapping its first two coordinates, thus obtaining an element of \(\mathbf{O}\). It is easy to see that each of these vectors has norm one:
$$v_i \cdot v_i = 1 $$But the diligent reader will also use this \(\mathrm{E}_8\) Dynkin diagram with its nodes numbered:
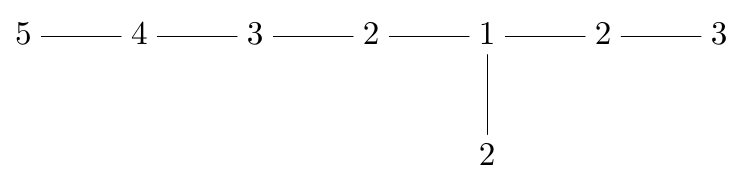
and verify that for \(i \ne j\) we have
$$ v_i \cdot v_j = -\frac{1}{2} $$when the corresponding dots in the diagram are connected by an edge, but
$$ v_i \cdot v_j = 0 $$otherwise.
Now we move up to 10 dimensions. We give \(\mathbb{R}^{10}\) a Minkowski metric where the first coordinate is timelike:
$$ (t, z, x_1, \dots, x_8) \cdot (t, z, x_1, \dots, x_8) = - t^2 + z^2 + x_1^2 + \cdots + x_8^2 $$With this metric we call \(\mathbb{R}^{10}\) "\(\mathbb{R}^{9,1}\)". We can identify \(\mathfrak{h}_2(\mathbb{O})\) and its Minkowski metric with \(\mathbb{R}^{9,1}\) as follows:
$$ \left( \begin{array}{cc} t + z & x \\ x^* & t-z \end{array} \right) \mapsto (t, z, x_1, \dots, x_8) $$Next, we make the \(v_i\) into vectors in \(\mathbb{R}^{9,1}\) by putting two zero components onto the front of each one. Their inner products are unchanged. Thus, if we can find two more unit spacelike vectors \(v_a, v_b \in \mathbb{R}^{9,1}\) with
$$ v_a \cdot v_b = -\frac{1}{2} $$ $$ v_b \cdot v_1 = -\frac{1}{2} $$and with all their other inner products with the \(v_i\) being zero, we will have a full set of (rescaled) simple roots for \(\mathrm{E}_{10}\) sitting inside \(\mathfrak{h}_2(\mathbf{O})\).
Here are two such vectors:
$$ \begin{array}{ccccccccccc} v_a &=& (0,& -1, & 0, & 0, & 0, & 0,& 0,& 0,& 0, & 0) \\ v_b &=& (\frac{1}{2} , & \frac{1}{2} , & 0, & -1, & 0, & 0, & 0,& 0, & 0, & 0) \end{array}$$It is obvious that \(v_a\) is orthogonal to \(v_1,\dots,v_8\), and not too hard to check that \(v_b\) is orthogonal to \(v_2,\dots,v_8\) and has \(v_b \cdot v_1 = -1/2\).
So, now we have rescaled copies of the simple roots for \(\mathrm{E}_{10}\), \(v_a, v_b, v_1, ..., v_8\), all contained in \(\mathfrak{h}_2(\mathbf{O})\). To complete the proof, it suffices to check that they are a basis for the lattice \(\mathfrak{h}_2(\mathbf{O})\). To be precise: integer linear combinations of these vectors give the whole lattice.
One basis for \(\mathfrak{h}_2(\mathbf{O})\) consists of the matrices
$$ \left( \begin{array}{cc} 0 & v_i \\ v_i^* & 0 \end{array} \right) $$for \(i=1,\dots,8\), along with two more matrices:
$$ \left( \begin{array}{cc} 1 & 0 \\ 0 & 0 \end{array} \right) $$and
$$ \left( \begin{array}{cc} -1 & 0 \\ 0 & 1 \end{array} \right) $$These two matrices correspond to the following vectors in \(\mathbb{R}^{9,1}\), respectively:
$$ \begin{array}{ccccccccccc} v_c &=& (\frac{1}{2} , &\frac{1}{2} ,& 0,& 0,& 0, & 0,& 0,& 0,& 0,& 0) \\ v_a &=& (0,& -1, &0, &0,& 0,& 0,& 0,& 0,& 0, &0) \end{array}$$So, we now have 10 vectors in \(\mathbb{R}^{9,1}\) that correspond to a basis of the lattice \(\mathfrak{h}_2(\mathbf{O})\): \(v_c, v_a\), and \(v_1, ..., v_8\) corresponding to the first eight matrices. The only difference between this basis and the rescaled \(\mathrm{E}_{10}\) roots is that this basis includes \(v_c\) rather than \(v_b\). But in fact we can write:
$$ v_b = v_c - 2v_1 - 3v_2 - 4v_3 - 5v_4 - 6v_5 - 4v_6 - 2v_7 - 3v_8 $$So the matrices that convert back and forth between the bases \(\{v_a, v_c, v_1, \dots, v_8\}\) and \(\{v_a, v_b, v_1, ..., v_8\}\) both have integer entries, and we are done. █
Of course, a more conceptual proof would be nice. I'm sure that will come in time. But for now I'm just basking in the warm glow of knowing this fact is true. It ties together a lot of things about the octonions, string theory in 10 dimensions, \(\mathrm{E}_{10}\), and cosmological billiards in 11-dimensional supergravity!
In the current proof, a lot of work happens at the very last step. Personally, I got mixed up when checking this step. If you want to verify that
$$ v_b = v_c - 2v_1 - 3v_2 - 4v_3 - 5v_4 - 6v_5 - 4v_6 - 2v_7 - 3v_8 $$or in other words
$$ -v_b = -v_c + 2v_1 + 3v_2 + 4v_3 + 5v_4 + 6v_5 + 4v_6 + 2v_7 + 3v_8 $$this should help:
$$ \begin{array}{ccccccccccc} - v_c&=& (-\frac{1}{2}, & -\frac{1}{2}, & 0, & 0, & 0, & 0, & 0, & 0, & 0, & 0) \\ 2 v_1&=&(0, & 0, & -1, & 1, & -1, & 0, & -1, & 0, & 0, & 0) \\ 3 v_2&=&(0, & 0, & 0, & 0, & 0, & 0, & 3, & 0, & 0, & 0) \\ 4 v_3&=&(0, & 0, & 0, & 0, & 2, & -2, & -2, & 0, & 0, & -2) \\ 5 v_4&=&(0, & 0, & 0, & 0, & 0, & 0, & 0, & 0, & 0, & 5) \\ 6 v_5&=&(0, & 0, & 0, & 0, & 0, & 3, & 0, & -3, & -3, & -3) \\ 4 v_6&=&(0, & 0, & 0, & 0, & 0, & 0, & 0, & 0, & 4, & 0) \\ 2 v_7&=&(0, & 0, & 1, & 0, & -1, & -1, & 0, & 0, & -1, & 0) \\ 3 v_8&=&(0, & 0, & 0, & 0, & 0, & 0, & 0, & 3, & 0, & 0) \\ - & - & - & - &- & - &- & - &- & - &- & - \\ -v_b &=& (-\frac{1}{2}, & -\frac{1}{2}, & 0, & 1, & 0, & 0, & 0, & 0, & 0, & 0) \end{array}$$Just add up all the vectors on top and check that you get the one at the very bottom! Of course you should also check that I wrote down these vectors correctly.
Theorem. Up to a rescaling, the \(\mathrm{E}_{10}\) lattice in 10-dimensional Minkowski spacetime is isometric to $$ \mathfrak{h}_2(\mathbf{O}) = \left\{ \; \left( \begin{array}{cc} a & b \\ b^* & c \end{array} \right) : \; b \in \mathbf{O} , \; a,c \in \mathbb{Z} \; \right\} $$ the lattice of \(2 \times 2\) self-adjoint integral octonion matrices in the space \(\mathfrak{h}_2(\mathbb{O})\) with its Minkowski metric.
Proof. This proof relies on two well-known but fairly heavy-duty facts:
We note that \(\mathfrak{h}_2(\mathbb{O})\) is the direct sum of the lattice $$ L = \left\{ \; \left( \begin{array}{cc} 0 & b \\ b^* & 0 \end{array} \right) : \; b \in \mathbf{O} \; \right\} $$ and the lattice $$ M = \left\{ \; \left( \begin{array}{cc} a & 0 \\ 0 & c \end{array} \right) : \; a, c \in \mathbb{Z}\; \right\} $$ All vectors in \(L\) are orthogonal to those in \(M\), so this is an orthogonal direct sum $$ \mathfrak{h}_2(\mathbf{O}) = L \oplus M $$ \(L\) is isometric to the lattice of Cayley integers, so as well-known (and shown in Egan's original proof) when we rescale it by a factor of \(\sqrt{2}\) we get the \(\mathrm{E}_8\) lattice in an 8-dimensional Euclidean space. When we rescale \(M\) by a factor of \(\sqrt{2}\), we get an even unimodular lattice \(\mathrm{H}\) in a 2-dimensional Minkowski spacetime.
So, after rescaling by a factor of \(\sqrt{2}\), \(\mathfrak{h}_2(\mathbf{O})\) is isometric to $$ \mathrm{E}_8 \oplus \mathrm{H} $$
A direct sum of even lattices is even, and the direct sum of unimodular lattices is unimodular. So, this direct sum is an even unimodular lattice in a 10-dimensional Minkowski spacetime, and thus
$$ \mathrm{E}_8 \oplus \mathrm{H} \cong \mathrm{E}_{10} $$
So, after rescaling, \(\mathfrak{h}_2(\mathbf{O})\) is isometric to \(\mathrm{E}_{10}\). \(\blacksquare\)
Note that we didn't really need that \(\mathrm{E}_8\) was the unique even unimodular lattice in 8d Euclidean space, but we did need the uniqueness for \(\mathrm{E}_{10}\).
The advantage of this proof is that it's short and slick. The advantage of the original proof is that it doesn't rely on heavy-duty results, and it gives us an explicit set of simple roots for \(\mathrm{E}_{10}\) sitting inside \(\mathfrak{h}_2(\mathbf{O})\). This will be nice when we try to apply this theorem.
You can also read comments on the n-Category Café, and make your own comments or ask questions there!

|
|

|