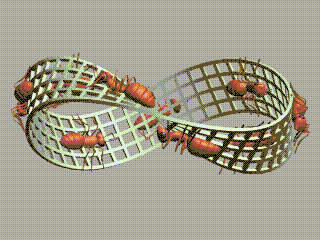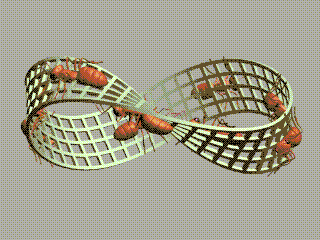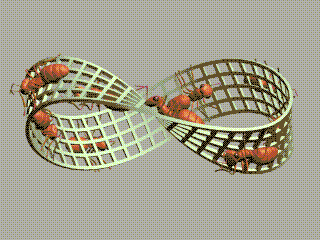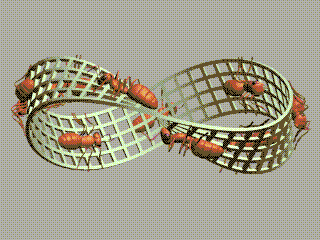
1 long short 2 small large 3 strong weak 4 sturdy flimsy 5 useful useless 6 beneficial harmful 7 bad good 8 imperfect perfect 9 dirty clean 10 pure impure 11 mixed simple 12 plain decorated 13 furnished bare 14 empty full 15 much little 16 long shortThe order of elements in each pair is random. We're going to order them.
Start with the first two pairs: long/short and small/large. Order the second pair to match the first! In other words, switch the order if large is more like long and small is more like like short. Otherwise, leave them alone.
Now ignore the first pair and look at the second and third pairs. Reorder the third pair to match the (possibly new) order of the second pair. And so on: go through the whole list and keep doing this.
I did this, and here's what I got:
1 long short 2 large small 3 strong weak 4 sturdy flimsy 5 useful useless 6 beneficial harmful 7 good bad 8 perfect imperfect 9 clean dirty 10 pure impure 11 simple mixed 12 plain decorated 13 bare furnished 14 empty full 15 little much 16 short long
We started with long/short and we ended with short/long! We've got a Möbius strip in the space of concepts!
Puzzle: why is this happening, and what does it mean?
Mathematicians know more precise way of saying what's going on... which doesn't answer the puzzle. We've got a double cover of the space of topics, where two sheets consist of two opposite adjectives. This double cover is nontrivial, because when we go around a loop the two opposites trade places. Using some math called 'homology theory', we can ask how many different ways this can happen.
The picture of ants on a Möbius strip goes back to Escher, but I found the animated gif here.
Tim Silverman writes:
Here's how I designed it to work:What are we doing when we compare these opposites? They don't have much in common semantically. But we do manage to classify them by some very general notion of "positive" vs "negative" and line up positives together and negatives together.
Actually, that was a lie... there are two general notions of "positive" vs "negative" in play. One covers stuff like quantity, dimension, number, intensity, etc. — "more" vs "less" in some very general sense. The other is evaluative: "better" vs "worse" — again in some very general sense.
And some words lie along one axis ("long" vs "short" is pretty much just more/less), some along the other (e.g. "good" vs "bad"), and lots are a mixture of both.and the two types of judgment don't need to align! E.g. with "strong"/"weak", more is better; but with "clean"/"dirty", "more" means "more dirt" — which is bad!
So we have a two-dimensional space, in which we can continuously rotate a vector to point in the opposite direction. So "long" and "large" are positive purely on the more/less scale; "strong", "sturdy", "useful" and perhaps even "beneficial" combine an increasing component of positive evaluation with a decreasing but non-zero degree of positive quantity or degree; "good" is purely evaluative; "perfect", "clean" and "pure" are all cases where the positively evaluated end of the scale is the one denoting an absence of something (imperfections, dirty, impurities); "simple" and "plain" are ambiguous (close to zero) on the axis of evaluation but clearly negative on the more/less scale and this holds true more strongly for "bare" and "empty"; then "little", "small" and "short" have very little or no evaluative component.
When I have the patience, I'll draw a picture.
The interesting thing is that when people are given the puzzle, they generally implicitly assume without question that the "positive" vs "negative" scale is 1-dimensional. Given this, there must be a discontinuity where the polarity switches, so to "understand" the puzzle, people look for the discontinuity — and they often think they've found it! They usually think it's somewhere around 3/4 of the way through, so maybe something is going on there, but I don't think it's the explanation of the puzzle.
I'm finally in a stable relationship!
When Lisa and I came to Erlangen, they put us in a really dreadful apartment in a boring little town nearby called Uttenreuth. This apartment had no soul, it had no privacy, the doorknob kept falling off... and we were not living in the charming city we'd expected; we had to take a bus to town. So we found another place. It's a house with two stories, but it's tiny: this is the whole upstairs, and downstairs there's a bedroom and bathroom. It used to be a stable!
We bump our heads on the ceiling... but the place has charm. It's on a quiet street called Loewenichstrasse, full of old buildings, right next to a park, but still just a 15 minute walk to the heart of town.
I'd been waiting for a couple weeks for a sunny day, so I could take a photo of this place. It was sunny this morning. Then it got cold again and started raining. But I didn't come to Germany for the weather. I came here for the Gemütlichkeit, the Weissbier and the Mohnschnecke! So I'm happy now.
Bullvalene has 10 carbon atoms (in black) and 10 hydrogens (in white). The cool thing about it is that these atoms are constantly rearranging themselves, quite quickly.
Molecules where some atoms easily change places are called 'fluxional molecules'. But what's special about bullvalene is that all ten carbon atoms trade places!
So, there are
different versions of bullvalene, and it keeps switching between these versions.
But since the molecule has 3-fold rotational symmetry, chemists say bullvalene has 10!/3 valence tautomers, where
For more, read this:
Though it's published by a chemistry society in a journal of chemistry education, they charge $35 to access this article for 48 hours... so students won't actually read it! This is an example of how the practice of science needs to be reformed. Luckily, you can get the article for free somewhere else... for now.
Luckily, you can also learn about bullvalene from Wikipedia:
There you can see the process that bullvalene uses to rearrange itself... and see related molecules like 'semibullvalene' and 'bullvalone'. Also try:
But here's a puzzle for physicists who know about bosons and fermions:
Puzzle: Identical atoms are indistinguishable, so what does it mean to say two traded places? How do we tell? Does this concept have any meaning?
Take a carbon and stick on 4 hydrogens: you get methane, the gas we like to burn. Yeah, I knew that. But take a carbon and stick on 5 hydrogens: then an electron falls off and you get methanium!
Huh?
Yes, it's a bit like ammonium. Take nitrogen and stick on 3 hydrogens, and you get ammonia. Stick on 4, an electron falls off and you get ammonium, a positively charged ion: NH4+. Similarly, methanium is CH5+.
But here's the cool thing about methanium. It's fluxional: it keeps changing. Usually three of the hydrogens form an equilateral triangle around the equator while the other two sit at the north and south pole. But now and then — in fact very often! — they trade places.
I recently told you about bullvalone, a fluxional molecule where all 10 carbons keep changing places. But I didn't tell you that they only change places if it's warm enough. When it's really cold, they lock into place.
What's cool about methanium is that it's fluxional no matter how cold it is. The uncertainty principle of quantum mechanics is enough to make the 5 hydrogens change places even at absolute zero!
You could say this is due to 'quantum zero-point energy', but I'm afraid to mention that, because then kooks will come out and tell us their schemes for exploiting this energy and saving the world.
Sorry, it doesn't work like that. It's just that even when methanium is at its lowest possible energy, when we can't possibly extract any energy out of it, the positions of the hydrogen atoms aren't fixed. If we keep measuring them they will change, thanks to the uncertainty principle. And they will change positions enough to actually trade places at a significant rate.
But the truth is cooler than fiction. Scientists have actually seen methanium in outer space, doing its thing.
For more see:
This picture is actually a glowing neon sculpture called "Methanium" made by a company called Wizard Glass
© 2014 John Baez
baez@math.removethis.ucr.andthis.edu