
Dallol is in Ethiopia near the Red Sea. It's the hottest place year-round on the planet, 125 meters below sea level, and it's geologically active. Intrusions of magma into ground water have repeatedly caused massive explosions, so the place is pocked with craters.
It's a highly dynamic place: active hot springs go inactive and new springs emerge from day to day, and their colors change from white to green, lime, yellow, gold, orange, red, purple and ochre.
These springs discharge hot brine that is more acidic than anywhere else on Earth: some other hot springs have a pH of less than 2, but here the pH is less than zero! The brine here is also anoxic: instead of oxygen, it contains carbon dioxide, hydrogen sulfide, nitrogen and sulfur dioxide. On top of that it's 10 times more salty than seawater, and it contains deadly concentrations of magnesium.
Can life survive here? In 2019 a team of scientists concluded that while the nearby salt plains are teeming with salt-loving microorganisms, there is no life in Dallol's brine ponds.
But then, that same year, another team found utra-small structures entombed in the mineral deposits — and identified them as very ancient organisms adapted to hypersaline environments! They're called Nanohaloarchaea.
More details and pictures:
There's also a ghost town nearby — once an active potash mine, and the hottest inhabited place on Earth.
On average the temperature here reaches 41 °C (105 °F) each day, and in June this goes to 47 °C (116 °F). Besides being hot, this area is also extremely dry, with only a couple days of rain a year.
This place would have beautiful in a desolate way if people hadn't messed it up.
I love this picture comparing different telescopes. Space telescopes are sexy — but the really big telescopes are down here. The Overwhelmingly Large Telescope is so big you might miss it: 100 meters across. Unfortunately the European Southern Observatory wimped out and decided to build a merely Extremely Large Telescope instead.
Radio telescopes are even bigger. The 305-meter dish at Arecibo is famous. In 2020 scientists decided to shut it down after it was damaged by a hurricane and two earthquakes and two important cables snapped. But before they could even shut it down, more cables snapped and the support structure, antenna, and dome assembly fell into the dish, destroying the whole thing.
Luckily in 2016 the Chinese had built an even larger radio dish in Guizhou: the Five-hundred-meter Aperture Spherical Telescope, or FAST. It's the largest one shown here. But in Russia there's a radio telescope 576 meters across, called RATAN-600 — not a dish, but a bunch of separate structures.
And then there's the xkcd cartoon....

Is this law true? In classical logic it is. But in intuitionistic logic it's not.
So, in intuitionistic logic we can ask what's the probability that a randomly chosen statement obeys the Law of Excluded Middle. And the answer is "at most 2/3 — or else your logic is classical".
This is a very nice new result by Benjamin Bumpus and Zoltan Kocsis:
Of course they had to make this more precise before proving it. Just as classical logic is described by Boolean algebras, intuitionistic logic is described by something a bit more general: Heyting algebras. They proved that in a finite Heyting algebra, if more than 2/3 of the statements obey the Law of Excluded Middle, then it must be a Boolean algebra!
Interestingly, nothing like this is true for "P implies not not P". They showed this can hold for an arbitrarily high fraction of statements in a Heyting algebra that is still not Boolean.
Here's a piece of the free Heyting algebra on one generator, which some call the Rieger–Nishimura lattice:
I disagree with this statement, but boy, Hilbert sure could write!
March 13, 2024
There's a lot we don't know. There's a lot we can't know. But can we at least know how much we can't know?
What fraction of mathematical statements are undecidable — that is, can be neither proved nor disproved? There are many ways to make this question precise... but it remains a bit mysterious. The best results I know appear, not in a published paper, but on MathOverflow!
In 1998, the Fields-medal winning topologist Michael Freedman conjectured that the fraction of statements that are provable in Peano Arithmetic approaches zero quite rapidly as you go to longer and longer statements:
He must also have been conjecturing that Peano Arithmetic is consistent, since if it's inconsistent then all its statements are provable. From now on let's assume that PA is consistent.
In 2005, Cristian Calude and Konrad Jürgensen published a paper arguing that Freedman was on the right track. More precisely, they showed that the fraction of statements of a given length in PA that are provable goes to zero as we go to longer and longer statements. The fraction of disprovable statements also approaches zero. So, the fraction of undecidable statements approaches 1.
Unfortunately their paper had a mistake!
In 2009, David Speyer argued that the fraction of provable statements does not approach 0 and does not approach 1 as we consider longer and longer statements. Instead, it's bounded by numbers between 0 and 1. Similarly for the fraction of undecidable statements! His argument is not air-tight, as he admits and explains---but I believe it. Someone should try to complete his proof.
Speyer's idea is very simple: if P is any statement, the statement "P or 1 = 1" is provable. This can be used to get a lower bound on the number of provable statements of a given length. Similarly, suppose G is some undecidable statement. Then for any statement P, the statement "G and (P or 1 = 1)" is undecidable. This can be used to get a lower bound on the number of undecidable statements of a given length.
March 14, 2024
There are only about 80,000 old growth giant sequoias left in California. After years of drought, roughly 10% of these enormous trees died in a massive fire in 2020. The future for them does not look good.
But I just learned that there are about 500,000 younger giant sequoias and closely related coastal redwoods in the UK!
They were first introduced in 1853 by the Scottish grain merchant Patrick Matthew. Later that year, the famous plant collector William Lobb brought over many more. Because of their rarity and novelty, these trees were very expensive. But that worked in their favor: they became a symbol of wealth in Victorian Britain. People planted them at the entrances of grand houses and estates, along avenues, and in churchyards and parks.
The map above shows just 4949 of the giant sequoias in the UK. Surprisingly, they thrive there, despite the climate being very different from that in their native range — the Sierra Nevada mountains, dry in the summer and snowy in the winter.
They are now the largest trees in the UK! A new study shows each one sequesters carbon at the rate of 85 kilograms per year, on average. This is very high for trees, and sequoias keep growing for centuries. People are wanting to plant more.
Invasive species — or wonderful rescue of a species that might otherwise go extinct? Evolution does its thing regardless of our value judgements, but what we do affects it. Long ago, giant sequoias were common in North American and Eurasian coniferous forests. By the last ice age, their range had shrunk to a small area in California. Now, thanks to humans, they are spreading again.
I got most of my information and paraphrased some of my sentences from here:
A bat, not scared by a carnivorous plant.
A pitcher plant found in Borneo uses an echo reflector to attract bats. But it's not trying to eat bats! The bat's poop provides the plant with nutrients. In return, the plant offers the bat a nice place to sleep — inside the pitcher.
This symbiosis is between a very specific pitcher plant, Nepenthes hemsleyana, and a very specific bat, Hardwicke’s woolly bat (Kerivoula hardwickii). However, if they can't find their favorite pitcher plant, desperate Hardwicke’s woolly bats will sometimes try other species, even the fanged pitcher plant Nepenthes bicalcarata. Yes, it's not just bats who have fangs! Details and pictures here:

What did Einstein do after discovering general relativity in 1915? Sit around and complain about the Lord playing dice with the universe? Not quite:
I think it's cool that items 3 and 4 served as the basis for current and future quantum technologies. He was always way ahead of his time.
And don't forget all his work on unified field theories! Though not
successful, it was crucial in lifting the goals of theoretical physics
to something very ambitious: unifying all the forces of nature! He
spent most of his later years on this.
March 27, 2024

Sometime this year, the star T Corona Borealis will go nova and become much brighter! At least that's what a lot of astronomers think. So examine the sky between Arcturus and Vega now — and look again if you hear this event has happened. Normally this star is magnitude 10, too dim to see. When it goes nova is should reach magnitude 2 for a week — as bright as the North Star. So you will see a new star, which is the original meaning of 'nova'.
But why do they think T Corona Borealis will go nova this year? How could they possibly know that?
It's done this before. It's a binary star with a white dwarf orbiting a red giant. The red giant is spewing out gas. The much denser white dwarf collects some of this gas on its surface until there's enough fuel to cause a runaway thermonuclear reaction — a nova!
We've seen it happen twice. T Corona Borealis went nova on May 12, 1866 and again on February 9, 1946. What's happening now is a lot like what happened in 1946.
In February 2015, there was a sustained brightening of T Corona Borealis: it went from magnitude 10.5 to about 9.2. The same thing happened eight years before it went nova the last time.
In June 2018, the star dimmed slightly but still remained at an unusually high level of activity. Then in April 2023 it dimmed to magnitude 12.3. The same thing happened one year before it went nova the last time.
If this pattern continues, T Corona Borealis should erupt sometime between now and September 2024. I'm not completely confident that it will follow the same pattern! But we can just wait and see.
This is one of only 5 known repeating novas in the Milky Way, so we're lucky to have this chance.
Here's how it might work:
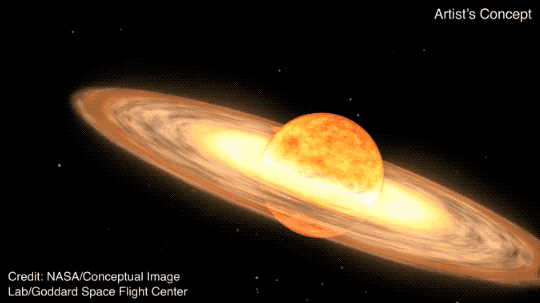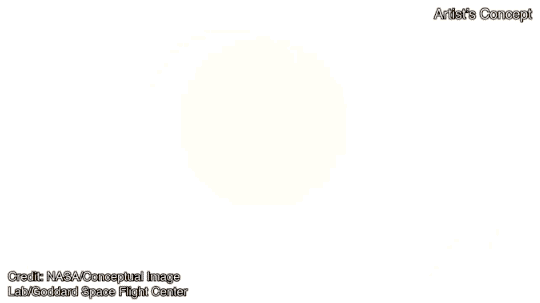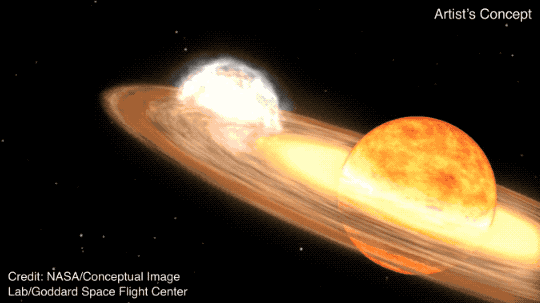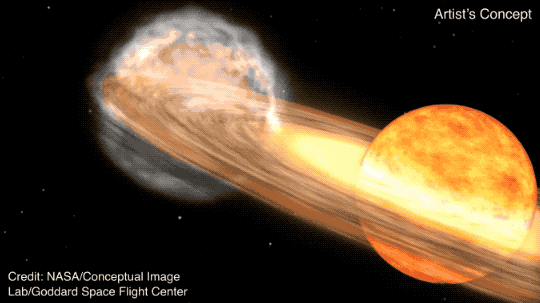
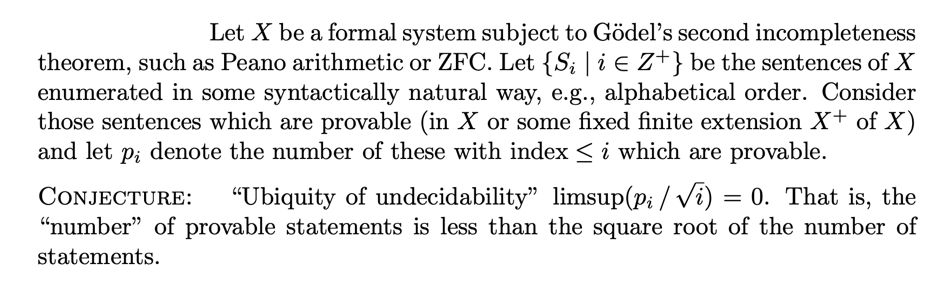
The description at NASA's blog:
A red giant star and white dwarf orbit each other in this animation of a nova. The red giant is a large sphere in shades of red, orange, and white, with the side facing the white dwarf the lightest shades. The white dwarf is hidden in a bright glow of white and yellows, which represent an accretion disk around the star. A stream of material, shown as a diffuse cloud of red, flows from the red giant to the white dwarf. The animation opens with the red giant on the right side of the screen, co-orbiting the white dwarf. When the red giant moves behind the white dwarf, a nova explosion on the white dwarf ignites, filling the screen with white light. After the light fades, a ball of ejected nova material is shown in pale orange. A small white spot remains after the fog of material clears, indicating that the white dwarf has survived the explosion.
For more details, try this:

Vancouver may soon see a dramatic addition. It's called Sen̓áḵw, and it consists of 11 tall towers holding 6,000 apartments. It’s being built by the Squamish First Nation. Since it's on land they own, they don't have to follow Vancouver’s zoning rules. And they've chosen to build bigger, denser and taller than anywhere else in Canada.
Predictably, this rubs against a widespread belief that the Squamish, as an indigenous people of the Pacific Northwest Coast, are living fossils with a duty to embody a romantic vision of life before Europeans came here.
City councillor Colleen Hardwick said “How do you reconcile Indigenous ways of being with 18-storey high-rises?” And Gordon Price, a Vancouver urban planner and a former city councillor, said “When you’re building 30, 40-storey high rises out of concrete, there’s a big gap between that and an Indigenous way of building.”
Neither of these people are members of the Squamish First Nation. Sen̓áḵw existed as a city of cedar longhouses long before Vancouver was built. Its Squamish residents saw their land carved up for railways. Then their houses were burnt down, and they were loaded onto a barge and shipped away. Now they're back.
Much of my text was paraphrased from this article, which features more cool pictures: