
The Wedding Palace in Ashgabat, Turkmenistan. From Atlas Obscura. This is one of many places it's fun to imagine visiting, that I don't feel any need to actually visit.
Raigad Fort, in Maharashtra, India. This became the capital of the Maratha Empire in 1674.
Red Clouds Golden Summit on Mount Fanjing in Guizhou, China.
Nemrut Dağ, the
"Throne of the Gods", in Turkey. This was the mausoleum of Antiochus
I (69–34 B.C.), who reigned over a kingdom north of Syria after the
breakup of Alexander's empire.
May 4, 2019
The aliens could be among us, and we'd never know!
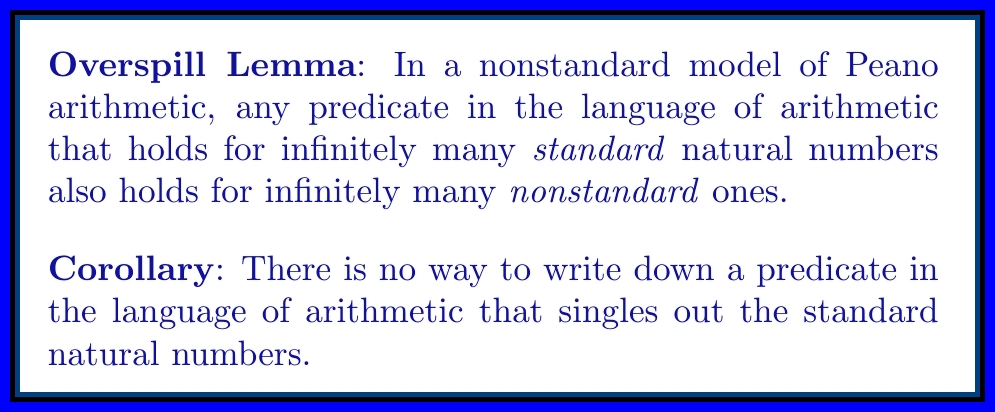
A 'nonstandard model' of the axioms of arithmetic contains extra 'nonstandard' natural numbers after all the usual ones. But you can't tell which ones those are! The Overspill Lemma makes this shocking fact precise.
Another corollary of the Overspill Lemma: in a nonstandard model of arithmetic, there are infinitely many nonstandard prime numbers, bigger than all the usual primes.
Nonstandard models of arithmetic are really cool! To learn a bit more without working too hard, a good place to start is Victoria Gitman's blog post. She's one of the best technical logic bloggers:
In the evening someone took a picture of us:
From left to right are Jason Erbele, Daniel Cicala, Christina Vasilakopoulou, me, Jeffrey Morton, Joe Moeller and Christian Williams. Unfortunately Kenny and Jade had already left.
You can see our talk slides here:
Yes! Take the proposition "true implies false", or \( \top \Rightarrow \bot\) in the notation of symbolic. Then:
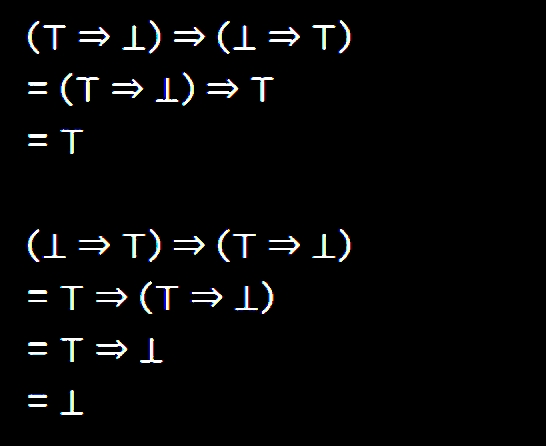
In other words, "'true implies false' implies 'false implies true'" is true,
but "'false implies true' implies 'true implies false'" is false.
May 26, 2019
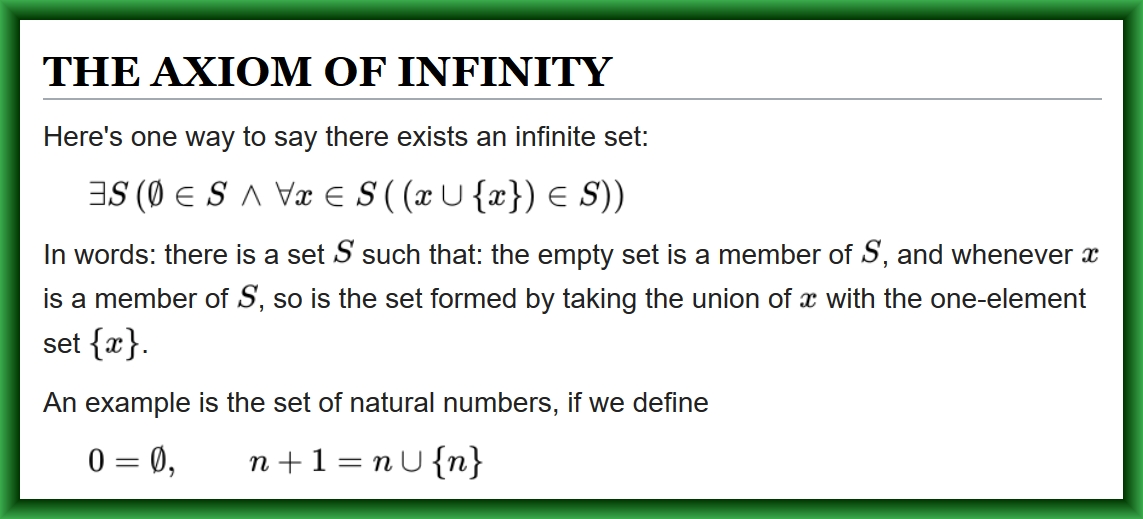
The Zermelo-Fraenkel axioms include an axiom saying there exists an infinite set. This is called the 'axiom of infinity'.
But what happens if we drop the axiom of infinity? We get a system called ZF-∞. These axioms are consistent if and only if the usual first-order axioms of arithmetic, PA, are consistent. One model of these axioms consists of all hereditarily finite sets. These are the sets with finitely members, all of which themselves are hereditarily finite. (Another axiom of ZF, the axiom of foundation, ensures that any descending chain — of members of members of members, etc. — must be finite.)
If we take ZF, replace the axiom of infinity by its negation, and add an axiom that permits a form of induction, then we get an axiom system that is 'definitionally equivalent' to PA. This implies that any model of PA gives rise to a model of these axioms, and vice versa.
Any model of ZF with the axiom of infinity replaced by its negation still contains infinitely many sets. But it has no infinite set: the collection of all sets is not a set: it's a proper class.
So even though this axiom system describes a world of infinitely many things, infinity is not a thing in this world.
For more details, see: