

This place is called the Cliffs
of Hathor. The "snow" is dust moving slowly; you can also see
some stars moving downward in the background, due to the rotation of
the comet.
November 2, 2018
Please read this report — it's important:
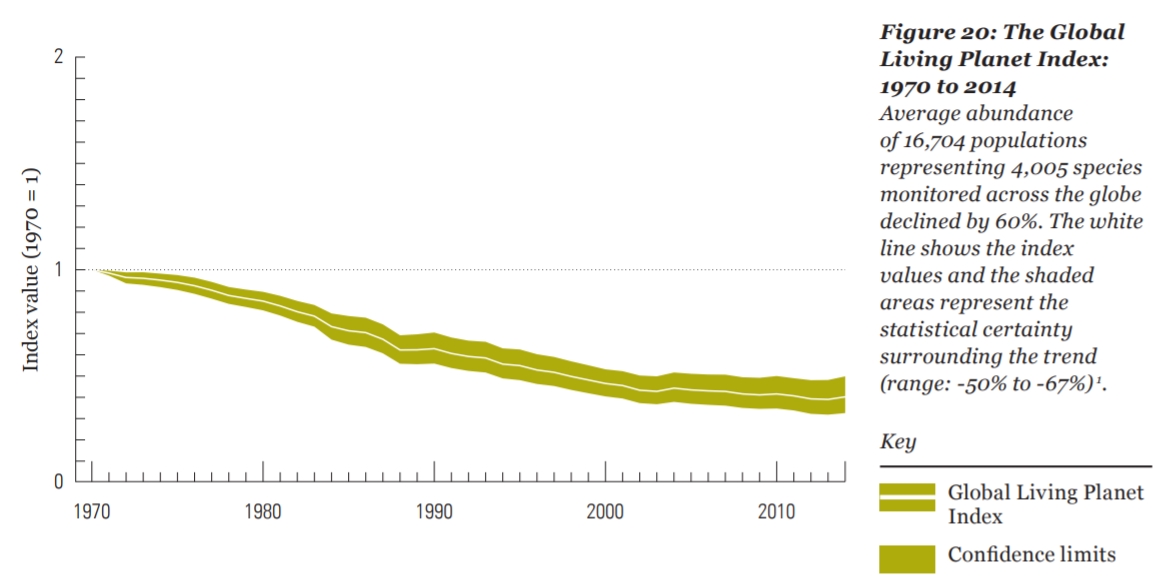
The situation is worse for freshwater species: they're down by about 83% since 1970:

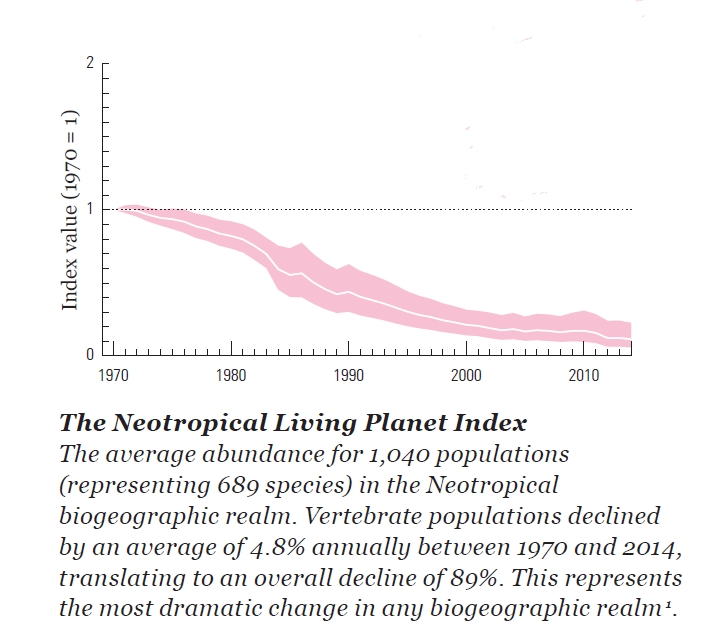
On land, the worst declines are occurring in the "Neotropical" region: Central and South America. Mammals, birds, reptiles and amphibian population have dropped by about 89% since 1970.
Slowly lower yourself toward the event horizon of black hole. As you do, look up. Your view of the outside universe will shrink to a point — and become brighter and brighter, tending to infinite brightness!
These effects don't happen if you simply let yourself fall in to the black hole. If you do that, your view of the outside world will not shrink to a point, and the light you see will not be intensified by blueshifting — because you'll be falling along with it!
Andrew Hamilton made this animated gif. See more here:
Good news: starting early next year, Eddie Bernice Johnson will be the first African-American to lead the House Committee on Science, Space, and Technology. She'll also be the first woman to do it. She's the first Democrat to lead this committee since 2011. She's the first who isn't a climate science denier since 2011. And she's the first with some training in science since the 1990s: she was chief psychiatric nurse at the Dallas Veteran's Administration Hospital for 16 years.
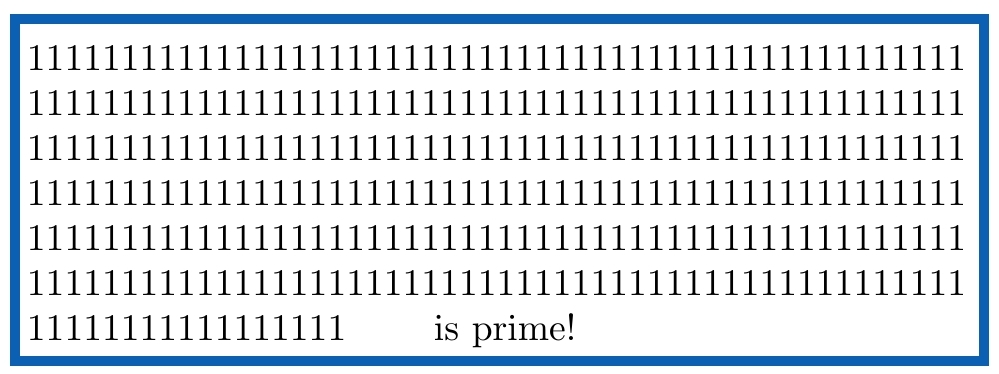
This number has 317 digits, all ones. It's prime. 317 is also prime!
That's not a coincidence. A number whose digits are all 1 can only be prime if the number of digits is prime!
This works in any base, not just base ten. Can you see the quick proof?
A prime whose decimal digits are all ones is called a "repunit prime". The largest known repunit prime has 1031 digits.

Mathematicians believe there are infinitely many repunit primes, but nobody can prove it yet.
Why does this matter?
The density of primes decreases slowly, like \(1/\ln n \). So, if repunits have 'no good reason not to be prime', the probability of finding an \(N\)-digit repunit prime should be roughly \[ \frac{1}{\ln 10^N} = \frac{1}{N \ln 10} \] Since the harmonic series diverges, this gives a probabilistic argument that there should be infinitely many repunit primes.
But what does probability really mean when it comes to prime numbers? God didn't choose them by rolling dice!
This is why silly-sounding puzzles about primes can actually be important: they challenge our understanding of randomness and determinism.
There might be infinitely many true facts about primes that are true just because it's overwhelmingly 'probable' that they're true... but not for any reason we can convert into a proof.
However, even this has not yet been proved.
Clouds of mystery surround us.
November 22, 2018
It's Thanksgiving!
I am thankful for the beauty of mathematics and physics, which always go deeper than I expect.
For example, Hamilton's equations describe the motion of a particle if you know its energy. But they turn out to look a lot like Maxwell's relations in thermodynamics!
Maxwell's relations connect the temperature, pressure, volume and entropy of a box of gas — or indeed, a box of anything in equilibrium. Nobody told me they're just Hamilton's equations with different letters and vertical lines thrown in.
So I decided to see what happens if I wrote Hamilton's equations in the same style as the Maxwell relations. It freaked me out at first. What does it mean to take the partial derivative of \(q\) in the \(t\) direction while holding \(p\) constant?
But it turns out to be okay. Indeed, this was a useful clue. I thought about it longer and realized what was going on.
You get equations like Hamilton's whenever a system extremizes something subject to constraints. A moving particle minimizes action; a box of gas maximizes entropy.
Read the details here:
Soon you'll never be bored. And if you get stuck and frustrated, just ask people. True seekers will be happy to help.
November 24, 2018
The game of "58 holes", or "hounds and jackals", is very ancient. Two players took turns rolling dice to move their pieces forward. This copy comes from Thebes, Egypt. It was made in the reign of Amenemhat IV, during 1814–1805 BC, in the Twelfth Dynasty of the Middle Kingdom. It's now at The Metropolitan Museum of Art.
But why 58 holes? That's a strange number!
The holes come in two groups of 29. Nobody knows the rules for sure! But the Russian game expert Dmitriy Skiryuk argued that the players move their pieces from holes A to 29 and then the large shared hole H, where they exit the board.
If so, each player really has 30 holes! That makes more sense: the number 60 was very important in Egypt and the Middle East. So "58 holes" is a red herring.
You can see Skiryuk's hypothesized rules, the above pictures, and more here:
The game was really widespread: here's one from a pillaged Iron Age tomb in Necropolis B at Tepe Sialk, Iran. It's now in the Louvre.
But here's something even cooler. The game was just found in Azerbaijan, almost 2000 kilometers from the Middle East — chiseled into a rock by Bronze Age herders!
Read more here:
I guess nobody can resist a good game. Someone should popularize this one again.