
© Copyright Akiyoshi Kitayoka 2009 March 3
This is not a spiral! See
This is the ceiling of the tomb of the famous Persian poet Hafez, who was born in the city of Shiraz in 1315, and died there in 1390. The current version of the tomb dates back only to 1935 and was designed by the French architect and archaeologist André Godard. But the design is beautiful!
There's a lot of fun stuff to see if you zoom in, but let's think about the stars.
Puzzle 1: How many points do the stars here have?
Puzzle 2: How many different kinds of stars are there with this many points?
For example, there's just one kind of 5-pointed star, but two kinds of 7-pointed star. There's a 7-pointed star with blunt points where you draw a line from each dot to the dot 2 after it, and one with sharp points where you draw a line from each dot to the dot 3 after it.
Puzzle 3: How many different kinds of n-pointed stars are there?
Puzzle 4: How many of these are connected?
For example, there is no connected 6-pointed star. If you take a regular hexagon and draw a line from each dot to the dot 2 after it, you get the traditional Star of David, which consists of two separate triangles. If you draw a line from each dot to the dot 1 after it, you get the hexagon... you can decide if that counts as a star. If you draw a line from each dot to the dot 3 after it, you get three straight lines meeting at the center... you can decide if that counts as a star. And that's all you can get, if you're following the rules I have in mind!
You can learn more here:
Hafez was a Sufi, and his poems show that:
This is from The Subject Tonight is Love, translated by Daniel Ladinsky. For more in translation, try:
This image is from 'Worldsday' at deviantart.
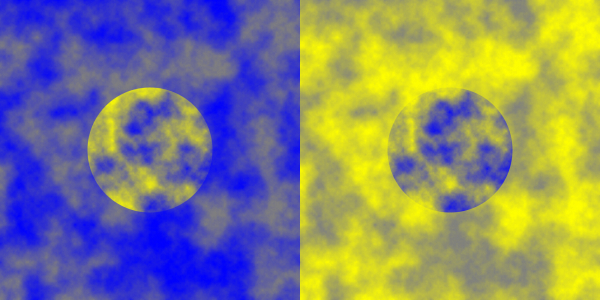
Context matters! The two disks are exactly the same in every way. Only their surroundings differ.
This is a metaphor for many things in life. What's the best example where the context of an event changed your perception of it?
For more great visual effects by the psychologist Akiyoshi Kitaoka, go here:

If you take every other corner of a cube, you get the corners of a demicube.
In 3 dimensions a demicube is just a regular tetrahedron! So you get two tetrahedra in a cube, as shown here. Together they form a stellated octahedron. In other words, you can also get this shape by taking a regular octahedron and sticking a tetrahedron on each of its faces — getting a kind of 3-dimensional star! It makes a great Christmas tree ornament.
What's a demicube in 4 dimensions? A 4-dimensional cube has 24 = 16 corners, so the demicube has 8. The cool part, special to 4 dimensions, is that all these corners point at right angles to each other, as viewed from the center of the cube!
If the corners of the 4-dimensional cube are
$$ (\pm 1,\quad \pm 1,\quad \pm 1,\quad \pm 1) $$
then we can get a demicube by taking those with an even number of minus signs. That gives these four:
$$ \begin{array}{lrrr} (1,& 1,& 1,& 1) \\ (1,& 1,& -1,& -1) \\ (1,& -1,& 1,& -1) \\ (-1,& 1,& 1,& -1) \end{array} $$
and their negatives. And if you know your math, you can check that the dot product of any two of the vectors I listed is zero! That means they all point at right angles to each other.
In fact, it means that the demicube in 4 dimensions is just the 4-orthoplex: the 4d analogue of an octahedron! We usually make a 4-orthoplex by taking these four vectors: $$ \begin{array}{rrrr} (1,& 0,& 0,& 0) \\ (0,& 1,& 0,& 0) \\ (0,& 0,& 1,& 0) \\ (0,& 0,& 0,& 1) \end{array} $$
and their negatives, and using those as corners. Each pair of the vectors listed is at right angles to each other. But the corners of a demicube work just as well, giving a 4-orthoplex that's twice as big in every direction, and rotated.
I don't know anything exciting to say about demicubes in 5 or 6 dimensions. But in 7 dimensions something very nice happens! A 7-cube has 27 = 128 corners, so a 7-demicube has 64. The 7-dimensional analogue of a tetrahedron, called a 7-simplex, has 8 corners. Notice: 64 is 8 times 8.
Can we take a 7-demicube and partition its corners into 8 sets of 8, each set being the corners of a 7-simplex?
Yes we can! Greg Egan figured out how.
The trick involves the Fano plane. This is a little gadget with 7 points and 7 lines, where any two points lie on a single line and any two lines intersect in a single point. If you haven't ever seen the Fano plane, what I just said is enough to draw it, so that might be fun to try... but beware: some of the lines will need to look curved if you draw it on an ordinary sheet of paper!
So, in 7 dimensions there's a picture like the one here, but with 16 different simplexes stuck inside a cube, instead of just two. That would be fun to see!
The next opportunity to partition the corners of a cube into simplices occurs in 15 dimensions.
Puzzle 1: can you take the set of 15-bit strings and find 16 of them, each pair of which agrees in exactly 7 places?
If you succeed, you'll have a 15-simplex inside the 15-cube, since you've taken the corners of a 15-cube:
$$ (\pm 1, \pm 1, \pm 1, \pm 1, \pm 1, \pm 1, \pm 1, \pm 1, \pm 1, \pm 1, \pm 1, \pm 1, \pm 1, \pm 1, \pm 1 ) $$
and found 16 of them, any pair of which has a dot product of -1. This is exactly what you need for the corners of a 15-simplex!
Moreover, since any pair of your bit strings disagrees in an even number of places (namely 8), your simplex will actually lie in a demicube.
If this puzzle was too easy, move on to:
Puzzle 2: can you take the set of 15-bit strings and partition it into sets of 16, such that any two strings in a given subset agree in exactly 7 places?
If so, you'll have found a way to partition the vertices of a 15-dimensional demicube into 15-dimensional simplices! 2048 of them, in fact.
After first raising these puzzles, I figured out how to solve them. It turns out that we can partition the corners of an \(n\)-dimensional cube into sets, each being the corners of a regular \(n\)-simplex, if and only if \(n\) is one less than a power of 2. And when \(n = 2^k - 1\) for \(k \ge 2\), each of these \(n\)-simplices lies in an \(n\)-dimensional demicube, so we can partition the vertices of the demicube into simplices.
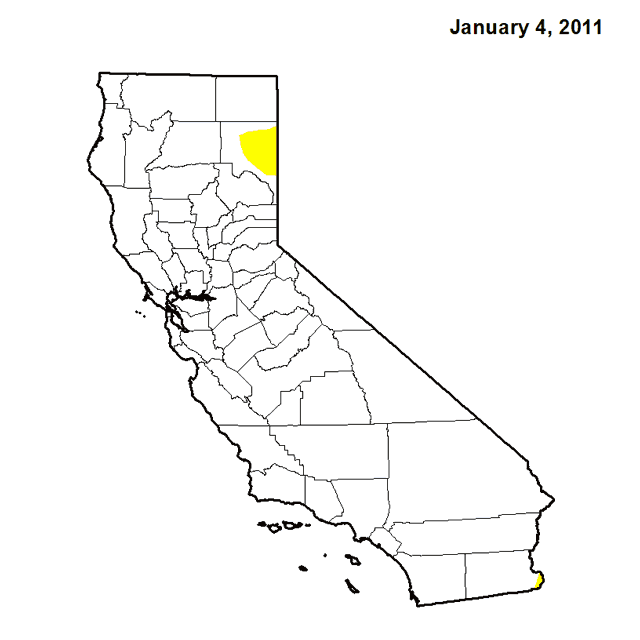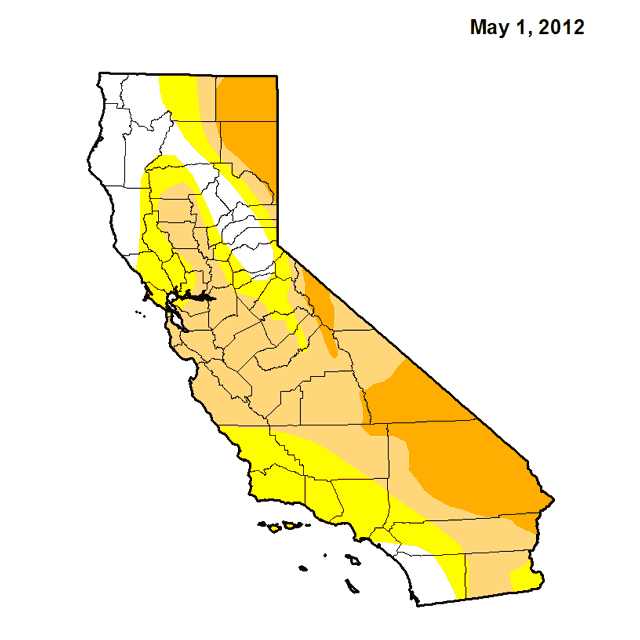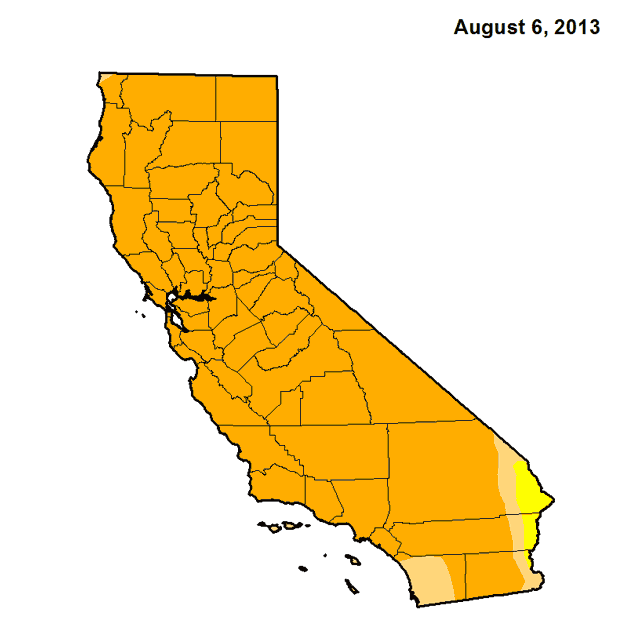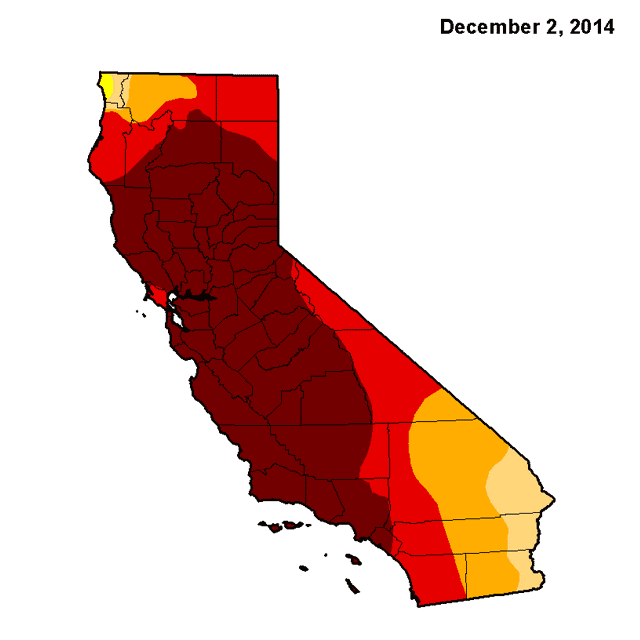
Down here in Southern California, we've had three good rains since the summer. Up north, they've gotten even more! In the first storm, ending December 3rd, San Francisco got more rain than they did all last year! They got 9.4 centimeters of rain in four days, compared to just 8.6 in 2013.
But we'd need a lot more rain to break the drought. It will take about 11 trillion gallons of water — 42 cubic kilometers — to fully recover from the drought. That's what researchers at NASA say, based on satellite data including measurements of the Earth's gravitational field, which depends on how much groundwater there is.
They say that since 2011, the Sacramento and San Joaquin river basins have decreased in volume by 4 trillion gallons of water each year: that is, 15 cubic kilometers. About two-thirds of the loss is due to depletion of groundwater beneath California's Central Valley:
Some scientists studying tree rings have claimed that as measured by the Palmer Drought Severity Index — a measure of precipitation and evaporation — this is the worst drought California has seen in 1200 years:
I would like to see the evidence, and the definitions involved — but I haven't seen them yet.
Dolphins blow rings of bubbles and play with them. Like smoke rings, these are examples of vortex tubes. You can also make vortex tubes that are knotted!
Long ago, the physicist Kelvin conjectured that for any kind of knot, you can create a vortex tube shaped like that knot. He even guessed that atoms were 'knotted vortex tubes in the aether'. That wasn't true, but his conjecture is still interesting - at least if we state it a bit more precisely.
Say you had a incompressible fluid with no viscosity. Then its velocity vector field would obey the Euler equations. The Euler equations have lots of steady solutions where the velocity of the fluid doesn't change with time. The fluid is still moving, but the same way all the time.
In these steady solutions, the fluid flows along curves. These curves can be very complicated!
Kelvin conjectured that for any knot, there's a stationary solution of the Euler equations where the fluid flows along a curve shaped like that knot.
This was recently proved to be true!
This paper will appear in the prestigious math journal Acta Mathematica. The paper is deep: in addition to a lot of work on topology and differential equations, it even uses some number theory! To get 'invariant tori' — that is, surfaces of vortex tubes — they use the Kolmogorov-Arnold-Moser theorem. This requires checking that some flow lines spiral around a torus with a slope that's an irrational number that's hard to approximate by rational numbers! This condition makes the torus robust against small perturbations.
Here's the abstract:
We prove the existence of knotted and linked thin vortex tubes for steady solutions to the incompressible Euler equation in \(\mathbb{R}^3\). More precisely, given a finite collection of (possibly linked and knotted) disjoint thin tubes in \(\mathbb{R}^3\), we show that they can be transformed with a \(C^m\)-small diffeomorphism into a set of vortex tubes of a Beltrami field that tends to zero at infinity. The structure of the vortex lines in the tubes is extremely rich, presenting a positive-measure set of invariant tori and infinitely many periodic vortex lines. The problem of the existence of steady knotted vortex tubes can be traced back to Lord Kelvin.A Beltrami field is a vector field with no divergence: $$ \nabla \cdot v = 0 $$ whose curl is proportional to itself: $$ \nabla \times v = c v $$ Any Beltami field gives a steady solution of Euler's equation!
William Irvine at the University of Chicago makes knotted vortex tubes in his lab, and this picture is from there.
Real-world fluids have viscosity, and then things get even more complicated and interesting. Vortex tubes can crash into each other and 'reconnect', as shown here!

Astronomers recently photographed this hole on Mars! There's no way to explain it by natural processes, and it's very regular in shape, so they believe it was produced by intelligent life. Since there's no life on Mars now, it must have been made by visitors from some other planet!
This hole is 1.6 centimeters across and 6.6 centimeters deep. It's in a rock in Gale Crater. It was drilled by the NASA rover Curiosity on May 19, 2013.
The rock, which NASA dubbed 'Cumberland', is interesting because it's made of ancient mud. NASA found that the ratio of deuterium to ordinary hydrogen in this rock is half the ratio seen in the water vapor in the Martian atmosphere. This suggests that Mars has lost a lot of water since the formation of Cumberland, probably about 3.6 billion years ago during the Hesperian Period — the period when Mars dried out and its atmosphere thinned to its current density.
Puzzle: Why would water on Mars have more deuterium now?
A bunch of the clay in Cumberland is 'smectite'. I had to look that up. Clay turns out to be quite interesting — like most other things, if you dig deep enough. Clay minerals are made of tetrahedral sheets of silica and octahedral sheets of hydroxide. There are two kinds: 1:1 clays and 2:1 clays. A 1:1 clay consists of alternating layers with one tetrahedral sheet followed by one octahedral sheet: examples are kaolinite and serpentine. A 2:1 clay consists of an octahedral sheet sandwiched between two tetrahedral sheets, and examples are talc, vermiculite and those in the smectite groups. I should include some pictures of these clay structures... maybe another day. For more on what they discovered by drilling this hole, read:
The photo is from this NASA webpage:
They're not really quantum, and they're not really ants — but they're cute, and Alexander Vlasov calls them qu-ants. Here's his explanation:
There are four states: 0 (empty, white), 1 (red), 2 (green), 3 (blue). A step may be divided into two stages:This is a cellular automaton. In other words, we've got a regular grid of cells, each colored from some finite set of colors, with a rule for updating all cells simultaneously based on the colors of their neighbors. But it's also reversible: the previous color of any cell before an update can be determined uniquely from the updated colors of all the cells. If you've got a reversible cellular automaton, you can run it backwards in time using another cellular automaton rule.First stage. Mark all cells satisfying two conditions:
1) the total number of red and blue cells in four closest positions is one or two
2) the cells in the four diagonal positions are either white (empty) or green.
Second stage. Change unmarked red cells to green, unmarked green cells to red, marked empty cells to red, marked red cells to blue, marked green cells to empty, and marked blue cells to green.
Vlasov actually constructed his qu-ants as a 'second-order cellular automaton'. This is a different kind of thing, where the color of each square depends on what's going on in its neighborhood in the previous two time steps. It's easy to make reversible second-order cellular automata... just like how Newton's laws of physics are reversible and given by second-order differential equations. But the description above conceals this fact, and describes the qu-ants as an ordinary cellular automaton.
For more information and more examples, see:
Water can freeze to form 'cages' that trap other molecules. They're called clathrate hydrates. There are several kinds, all beautiful. Nature is a great geometer!
This image, animated by Isaac Calder, shows a type I clathrate. The oxygen atoms in the water are at the corners of 12-sided and 14-sided shapes.
The 12-sided shapes have pentagons as sides, but they are not regular dodecahedra — if you look carefully, you'll see the pentagons are a bit off. The 14-sided shapes also have two hexagonal sides!
All this is very similar to the Weaire-Phelan structure, the best known solution to an old puzzle raised by Kelvin. He asked how space could be partitioned into cells of equal volume with the least area of surface between them. He proposed a solution, and for a long time people thought it was the best possible, but in 1993 Weaire and Phelan found one where the area is 0.3% less. It looks a lot like this, but the surfaces are curved.
For a great explanation of different clathrate structures, go here:
It's worth learning how to enable Java applets just to see these clathrates in motion! Nowadays Windows makes it really hard to use Java applets that aren't registered in a certain way. But you can still do it.
Here's another view of a type I clathrate:

This photo is almost unbearably cute!
It was taken by Barry Bland at TIGERS — The Institute for Greatly Endangered and Rare Species, in Myrtle Beach, Florida.
It's interesting to think about why this photo is so cute.
First of all, obviously, the young wolf and tiger seem like pals, walking in step — and the wolf is even smiling! But more deeply, I think we like the idea that animals of different species, even fierce ones, could be friends. The lamb may not lie down with the lion, but at least the tiger can play with the wolf! It gives us hope.
Finally, these are young animals, and thus more friendly, playful and inquisitive than their adult versions... and more cute. We seem to be innately fond of baby animals, perhaps thanks to our instinct to care for human babies.
Dogs are neotenized wolves — adult dogs, especially of certain breeds, resemble young wolves, not only in looks (a more round head, etcetera) but in behavior. Dogs are now considered to be the same species as wolves, just a different subspecies. We clearly got along best with wolf puppies that stayed friendly and submissive.
We may ourselves be neotenized apes. It could be that intelligence, playfulness and curiosity are traits of youth that proved, in certain social contexts, to be adaptive even for adults. If so, there could be something profound about 'cuteness'. Perhaps our attraction to youthful, friendly, playful things helped spawn art, music, science, more merciful codes of morality, and more.
For more, see:

Is this a clever painting on the wall of a building? No, it's a view from the parking lot at Meteor Crater, in Arizona. Looking to the northwest, you see a number of distant mountains.