
This surface is beautiful — but it's also the best known solution to a hard math problem. It's called the Endrass octic.
Why 'Endrass'? Because was discovered in 1995 by Stephan Endrass while he was writing his Ph.D. thesis.
Why 'octic'? Because it's described by a polynomial equation of degree 8.
You'll notice it has lots of points where the tips of two cones meet. It has 168 of them, though not all are visible here. And this is, so far, the largest number of such points that people have gotten for an octic.
It may not be the best possible. But it's the best so far. In 1984, a guy named Miyaoka showed that you can't get get more than 174 of these conical points in an octic. So, there's a gap between what we know is possible and what might be possible. (If you're into algebraic geometry you might like Miyaoka's paper — he used some pretty fancy techniques.)
Endrass actually found two octics with 168 conical points. You can see pictures of both, drawn by Abdelaziz Nait Merzouk, over at my blog:
They're very beautiful. You can also see the equations of these surfaces. Those are not very beautiful, at least not to me. Endrass found them with the help of a computer algebra system.
The animated gif above was made by Abdelaziz Nait Merzouk. It's copyrighted under a Creative Commons Attribution-ShareAlike 3.0 Unported license.
Here's another depiction of the Endrass octic, from a German math website:
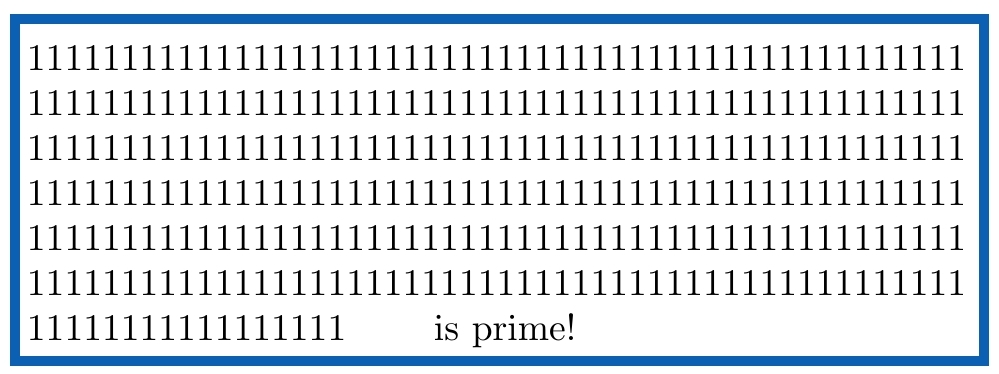
This is a prime number whose decimal digits are all ones. It has 317 ones. It's not the world record. The number with 1031 ones is also known to be prime!
Even larger guys like this are suspected to be prime. Are there infinitely many? Mathematicians believe so, but they can't prove it.
Why do they believe it? The main reason is that they have an estimate of the 'probability' that a number with some number of digits is prime. We can use this to guess the answer to this puzzle.
Of course the whole idea of 'probability' is a bit weird here. A number is either prime or not: the math gods do not flip coins to decide which numbers are prime!
Nonetheless, treating primes as if they were random turns out to be useful. Mathematicians have made many guesses using this idea, and then proved these guesses are right, using a lot of extra work.
Of course it's subtle. If I wrote down a number with 317 twos in its decimal expansion, you'd instantly know it's not prime — because it would be even.
In the European Congress of Mathematics, a number theorist named James Maynard just announced something cool. There are infinitely many prime numbers with no sevens in their decimal expansion!.
And his proof works equally well for any other number: there infinitely many primes without 0 as a digit, or 1, or 2, or 3, or 4, or 5, and so on.
This is big news, but not because mathematicians really care about primes with no sevens in them. It's because proving something like this requires a deep and delicate understanding of 'the music of primes' — the way prime numbers are connected to wave patterns. For more on that, here's something easy to read:
Thanks to Luis Guzman for pointing out this article, and thanks to David Roberts for finding James Maynard's paper on this subject, which is here:
He shows that if your base \(b\) is sufficiently large, you can find infinitely many primes that are lacking a chosen set of digits, where this set can contain up to \(b^{23/80}\) of the digits. Unfortunately I don't see how large b must be — he may not have worked this out. If \(b = 10\) counts as sufficiently large, then since \(10^{23/80}\) is about 1.94, this result would let you avoid any one digit in base 10, but not two. In any event, he does prove, separately, that you can find infinitely many primes that avoid any one digit in base 10.
It uses cool techniques, like "decorrelating Diophantine conditions which dictate when the Fourier transform of the primes is large from digital conditions which dictate when the Fourier transform of numbers with restricted digits is large". It also uses ideas from Markov process theory — that is, the theory of random processes — as well as hard-core number theory concepts.
By the way, a number whose decimal digits are are 1 is called a repunit. Here's a cool fact: the repunit with \(n\) digits can only be prime if \(n\) is prime. This is easy to see using an example. Consider the repunit with 35 digits: $$ 11111111111111111111111111111111111 $$ Since \(35 = 5 \times 7\), this repunit is not prime, and here's why: $$ 11111111111111111111111111111111111 = 11111 \times 1000010000100001000010000100001 $$ See? That's five 1's, times a number with 1 every fifth time and a total of seven 1's. If you imagine multiplying these out, you'll see why it works.
We can also factor the same number another way: $$ 11111111111111111111111111111111111 = 1111111 \times 10000001000000100000010000001 $$ That's seven 1's, times a number with 1 every seventh time and a total of five 1's.

We thought we knew. New measurements say we were 4% off. That may not seem like much — but it's enough to be a serious problem!
We can measure the proton radius by bouncing electrons off it, or by carefully studying the energy levels of a hydrogen atom. People have measured it many times, and the different measurements agree pretty well. Here's the answer:
A femtometer is \(10^{-15}\) meters, or a quadrilionth of a meter.
But you can make a version of hydrogen with a muon replacing the electron. The muon is the electron's big brother. It's almost the same, but 207 times heavier. So, muonic hydrogen is about 1/207 times as big across. And that makes the effects of the proton radius easier to detect!
So, in principle, we should be able to measure the radius of a proton more accurately using muonic hydrogen.
So that's what they did — in Switzerland, back in 2010. They repeated the experiment in 2013. Here's what they got:
In theory, this is about ten times more accurate. However, it's way off from all the earlier measurements! 7 standard deviations off, in fact.
This story is in the news again today. The same team just used muons to measure the radius of deuterium — a proton and a neutron stuck together. And again, they're getting a different answer than what people get using electrons.
Could some new physics be responsible? Some serious mistake in our theory of particles? The guy who led the new experiment, Randolph Pohl, said:
That would, of course, be fantastic. But the most realistic thing is that it's not new physics.
I like that. A good experimentalist does not jump to dramatic conclusions. Pohl guesses that we're wrong about the value of the Rydberg constant, a number that goes into calculating the proton mass from the experimental data. Another possibility, of course, is that he and his team are making some systematic error. It would be nice to have some completely different group of people check their results.
However, it's worth noting that there's another puzzle about muons. Electrons and muons are like tiny magnets. When we calculate how strong the magnetic field of an electron is, we get results that match experiment incredibly well. But when we do the same calculation for the muon, we're off by 3.4 standard deviations.
So maybe, just maybe, there's something funny going on with muons, which hints at new physics beyond the Standard Model. I doubt it. But you never know.
Check out this for more:
If our estimate of the Rydberg constant were 4 standard deviations off, that would do the job. That sounds like a lot... but if you look at the graphs here, you'll see other cases when we were way off about things!
For even more, check out this:
On Thursday, NASA will announce a planet orbiting Proxima Centauri — the star closest to our Sun, 4.24 light years away. They're trying to make this planet sound like Earth... and that's cool. But I'll tell you some ways it's not.
Mainly, Proxima Centauri is really different from our Sun!
It's a red dwarf. It puts out just only 0.17% as much energy as our Sun. So any planet with liquid water must be very close to this star.
And because it's cooler than the Sun, Proxima Centauri mainly puts out infrared light — in other words, heat radiation. Its visible luminosity is only 0.005% that of our Sun!
So if you were on a planet as warm as our Earth orbiting Proxima Centauri, it would look very dim — about 3% as bright as our Sun.
Of course, if there's life on this planet, it would probably evolve to see infrared.
But there's a more serious problem. Proxima Centauri sometimes puts out big flares, with lots of X-rays! That's not very nice.
Why does a wimpy little red dwarf have bigger flares than the Sun?
The Sun has a core where fusion happens, and helium produced down in the core mainly stays there. A red dwarf doesn't have a core: it's fully convective. In other words, heat moves through the star not by radiation, but by hot gas actually moving up to the surface.
All this ionized gas moving around makes big magnetic fields. The magnetic field lines get twisted up and sometimes explode out in flares! These flares get so hot that they emit X-rays. That's very hot.
Our Sun has flares too, but on a smaller scale. Even on a calm day, Proxima Centauri puts out as much X-ray energy as our Sun. But when a big flare occurs, it can put out 10 times more. This happens pretty often.
So: any 'Earth-like' planet orbiting this star will be a lot closer than the Earth is to our Sun, and get a lot more X-rays.
Puzzle 1. Use what I've told you to estimate how much closer a planet must be, to be at the same temperature as the Earth.
Puzzle 2. Estimate how much more X-rays it will get.
On top of this, Proxima Centauri could be part of a triple star system!
The closest neighboring stars, Alpha Centauri A and B, orbit each other every 80 years. One is a bit bigger than the Sun, the other a bit smaller. They orbit in a fairly eccentric ellipse. At their closest, their distance is like the distance from Saturn to the Sun. At their farthest, it's more like the distance from Pluto to the Sun.
Proxima Centauri is fairly far from both: a quarter of a light year away. That's about 350 times the distance from Pluto to the Sun! We're not even sure Proxima Centauri is gravitationally bound to the other stars. If it is, its orbital period could easily exceed 500,000 years.
On the bright side, Proxima Centauri will last a lot longer than our Sun. As it ages, it will get smaller and hotter, gradually changing from red to blue. After about four trillion years it will grow to 2.5% of the Sun's luminosity. When its hydrogen is exhausted, it will then become a white dwarf, without ever puffing up into a red giant like our Sun.
So, any planet orbiting this star will be a weirdly different world. But if we ever get there, we could stay for trillions of years, long after our Sun has become a red giant, roasting life on Earth.
For rumors of NASA's announcement, see this:
For answers to the puzzles, see my G+ post.
August 18, 2016
We live in a world of shadowy struggles. A team of hackers called the Equation Group has remarkable powers:
They also seem to be connected to StuxNet, the computer worm that destroyed centrifuges used by the Iranians for uranium enrichment.
Given all this, it's a good guess that the Equation Group is closey connected to the NSA, the National Security Agency of the US. I sort of hope so — because while that's scary, the alternatives scare me more.
Now another mysterious group called Shadow Brokers has released 256 megabytes of hacking tools that may be used by the Equation Group — and has offered to sell the rest for $500 million! They wrote:
We follow Equation Group traffic. We find Equation Group source range. We hack Equation Group. We find many many Equation Group cyber weapons. You see pictures. We give you some Equation Group files free, you see. This is good proof no? You enjoy!!! You break many things. You find many intrusions. You write many words. But not all, we are auction the best files.
At first researchers doubted that these guys had been able to steal software from the Equation Group. But new research at the cybersecurity firm Kaspersky Labs seems to confirm it.
Read this for more:
On how the Equation Group was found by researchers at Kapersky Labs last year:
On how Shadow Brokers released 256 megabytes of hacking software on their blog:
Today we're half-way through Hungry Ghost Month here in Singapore! This is the time when ghosts and spirits of deceased ancestors come out from the lower realm... and pester us if we haven't been feeding them with enough offerings.
Today seems to have been the main festival day, the Ghost Festival. There was a lot of fun stuff happening today in the local market! People are giving offerings to the kings of the underworld:
People are praying and giving offerings to their ancestors:
There were monks in white robes walking around. And there was live music — drums, clackers, cymbals and a horn!
But as usual, the market was also selling lots of food to the hungry people out there, like Lisa and me:
So we had Assam laksa for breakfast and enjoyed the music and ceremonies, then did our grocery shopping.
Here's some Assam laksa made by Bee Yinn Low, who has a nice blog on Malay food:
A staple — and arguably the most famous — hawker food in Penang, Penang Assam laksa is very addictive due to the spicy and sour taste of the fish broth. Tamarind is used generously in the soup base and hence the word Assam (means tamarind in Malay). In addition to tamarind, assam keping or peeled tamarind is also commonly added to give it extra tartness. Another secret ingredient is Polygonum leaf (marketed as Vietnamese mint leaf in the United States) or daun kesom/daun laksa. While the best Assam laksa broth is infused with the aromatic ginger flower (bunga kantan), I made without it because I couldn't find this special ingredient in the market. Of course, no Assam Laksa is complete without belacan and dollops of heh ko/prawn paste (the dark paste on the spoon).
The stuff we had was quite different — no Vietnamese mint, more
sardines, really fat white noodles — but it still had that sweet
sour flavor of tamarind. Very good! And very different than the
usual Singaporean laksa, which is made with coconut milk.
August 27, 2016
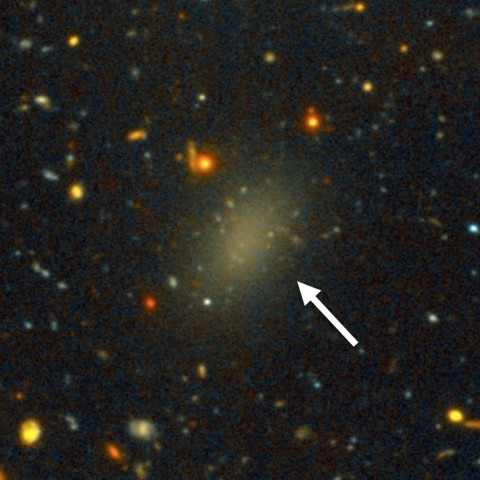
This week some astronomers published an exciting paper: they found a galaxy that's 98% dark matter.
It's called Dragonfly 44. It's extremely faint, so it doesn't have many stars. But we can use redshifts to see how fast those stars are moving — over 40 kilometers per second on average. If you do some calculations, you can see this galaxy would fly apart unless there's a lot of invisible matter providing enough gravity to hold it together. (Or unless something even weirder is happening.)
Something similar is true for most galaxies, including ours. What makes Dragonfly 44 special is that 98 percent of the matter must be invisible. And this is just in the part where we see stars. If we count the outer edges of the galaxy, the halo, the percentage could rise to 99% or more!
For comparison, the Milky Way is roughly 90% dark matter if you count the halo. We know this pretty well, because we can see a few stars out in there and measure how fast they're moving.
There are also galaxies like Messier 105 that may have less than the average amount of dark matter in their halo, though this is debatable.
And most excitingly, sometimes clusters of galaxies collide and stop moving, but their dark matter keeps on going!
We can see this because light from more distant galaxies is bent, not toward the colliding clusters, but toward something else. The most famous example is the Bullet Cluster, but there are others.
All these discoveries — and more — make dark matter seem more and more like a real thing. So it's more and more frustrating that we don't know what it is. As I explained a while ago, recent experiments to detect particles of dark matter have failed. So it could be something else, like black holes about 30 solar masses in size. And intriguingly, the first black hole collision seen by LIGO involved a 35-solar-mass and a 30-solar-mass black hole. These are too big to have formed from the collapse of a single star. They might be primordial black holes, left over from the early Universe.
But more on that later.
For more on Dragonfly 44, see:
For our failure to find dark matter particles, see my July 31 diary entry. For more on dark matter on the outer edges of galaxies, see:
For the Milky Way's dark matter halo, see:

Dolphins do this. Why? Maybe just for fun. But people actually debate this question. Here's what they say at Dolphins-World:
Why do dolphins jump out of the water?There is an ongoing debate about why dolphins jump out of the water. Scientists think about different reasons for this behavior.
Among them, some think that dolphins jump while traveling to save energy as going through the air consume less energy than going through the water.
Some other think that jumping is to get a better view of distant things, mainly food. So, in this way, dolphins jump to locate food or food related activity like seagulls eating or pelicans hunting.
Other explanation suggest that dolphins use jumping to communicate either with a mate or with another pod.
Some people even think that dolphins jump for cleaning, trying to get rid of parasites while jumping.
Finally some scientists think that they are only having good fun, as playing helps to keep senses at their best.
The idea that this double flip "saves energy" would be idiotic. The idea that they can find prey better by jumping out of the water than by using their sonar underwater also seems implausible. The other ideas are possible. But I think it's likely that all sufficiently intelligent life forms do stuff "just for fun". There are plenty of good biological reasons for this, I think.
And if you've ever seen the amazing games dolphins play with air bubbles, you'll know what I mean. If you haven't, check this out:
Greg Egan and I spent a lot of time working on 'topological crystals', but at some point he decided not to be a coauthor of the paper we were writing. So I finished it up myself, and now you can read about the idea here: