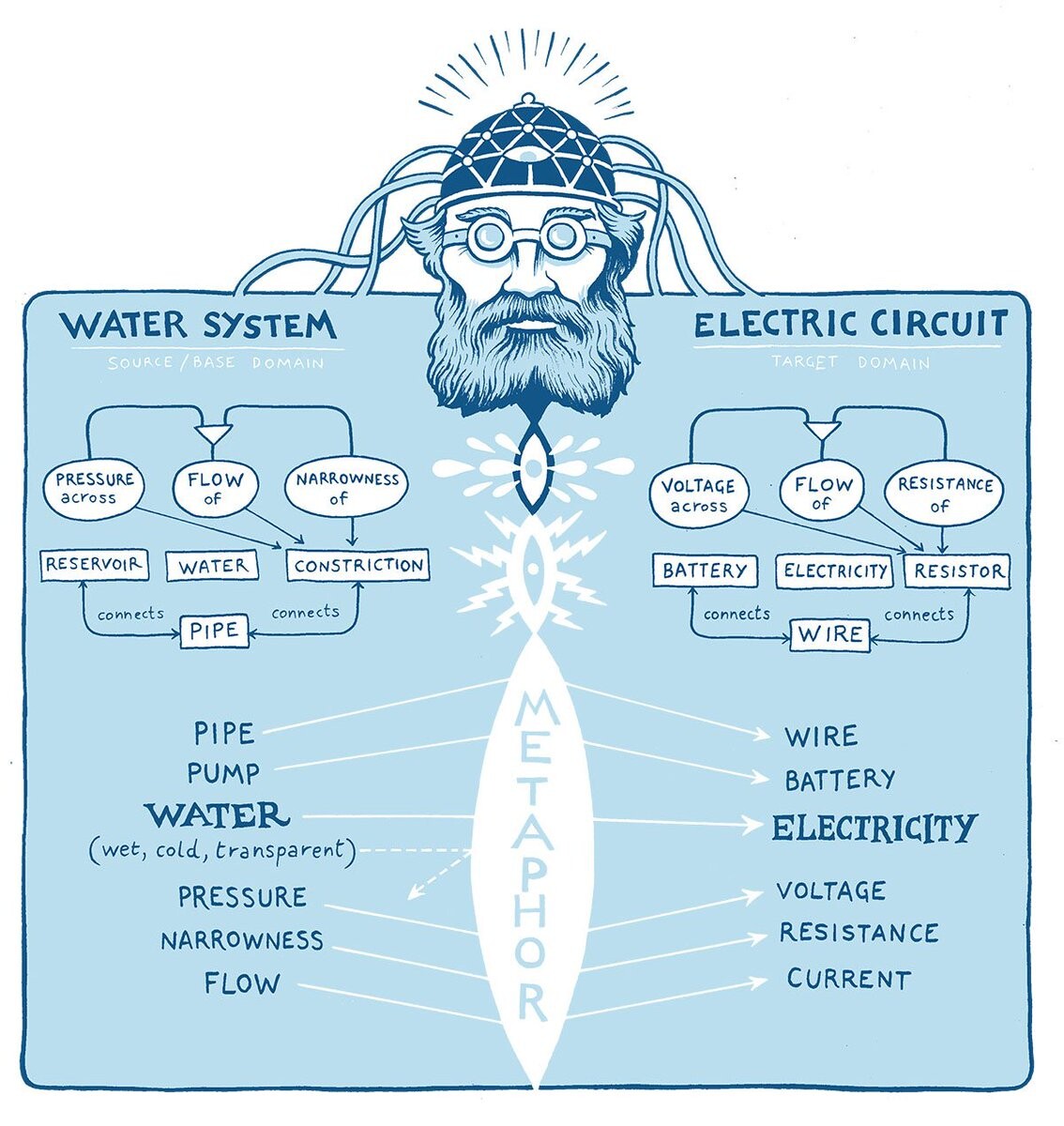

When you first realize that two seemingly different subjects are isomorphic, your neurons tingle — you feel like you're acquiring a new superpower!
I like this blissed-out dude in Matteo Farinella's book Of Microscopes and Metaphors: Visual Analogy as a Scientific Tool. Maybe he's a steampunk version of James Clerk Maxwell? Maxwell was big on the analogy between hydraulics and electromagnetism:
The notes in the major scale are spaced in a funny way. Look at the white keys on the piano: some have black keys between them, other don't. But you can understand the major scale using the circle of fifths. Start with C. Go up a fifth and you get G. Go up another fifth and you get D. Go up another and you get A. Go up another and you get E. Go up another and you get B. Go up another and you get F. And these are the notes in the C major scale!
That sounds like satisfying explanation. But it's a lie!
I lied only at the end. When you go up a fifth from B, you don't get F. You get F♯. So you don't get the notes in the major scale. You get the notes in another scale, called the Lydian mode! You can see it here:
This is why George Russell argued that Lydian is more fundamental than the major scale.
Russell is the theorist who helped Miles Davis switch to a new style of jazz in Kind of Blue—the best-selling jazz album of all time. In his book Lydian Chromatic Concept of Tonal Organization, Russell tried to redo harmony theory from the ground up. For a good explanation of Russell's ideas, watch this video:
And here's another piece of evidence for this crazy theory that Lydian is secretly more major than major, like the power behind the throne. If you take the 7 modern modes and order them by 'brightness', the Lydian mode is the brightest of all, while Ionian---that is, major---comes in second.
What's 'brightness'? Basically it's a measure of how high the notes are in a scale that starts with a fixed note, like C. To get from Lydian to Ionian you lower the fourth a half-step, turning F♯ to F in this chart:
To get from Ionian to Mixolydian you lower the seventh a half-step, turning B to B♭. And it keeps going like that: you keep flatting more notes, going to 'darker' modes.
In bright modes the notes are 'pushed up'. In dark modes the notes are 'pushed down'. Once you listen enough you can hear the difference. Bright modes aren't always 'happier', but they are higher, more 'celestial'. Dark modes aren't always 'sadder', but they are further down.
Another fun thing about Lydian is that the two half-steps occur right before the 5 and the 1. The half-step before the 1 is important in classical music: it's called the 'leading-tone' because there's an intense pressure to go up a half step from that note to the 1, which brings a sense of rest: this has been the real motor of Western music ever since the Renaissance. The 5 is the second most important note after the 1, since their frequency ratio is 3/2, which is a really simple fraction. So, having a half-step right before the 5 is almost like having another leading tone, given the importance of the 5.
Anyway, these patterns are fun to think about. But please don't think I've gone off the deep end. Lydian is not more important than major in modern Western music. It's used less often, and there's a damn good reason. That F I keep talking about is a perfect fourth above C. The major scale has that perfect fourth, Lydian doesn't. And that fourth is really important in modern Western harmony!
You could say the fourth and fifth are the 'backbone' of modern Western harmony. They provide the simplest vibrational frequencies: the fifth vibrates 3/2 times as fast as the 1, while the fourth vibrates 4/3 times as fast. 4/3 is one octave above 2/3. So if you ignore octaves, as we often do in harmony theory, going a fifth down is the same as going a fourth up.
Anyway, back to Lydian. I was pretty excited about the mathematical virtues of the Lydian mode, but then Mark Reid on Mastodon told me two things that led to a chain of realizations that really blew my mind. The first was this:
Something about Lydian I only realised recently is made very clear by that diagram above.Suppose, in the top row, you treat F♯ as a “natural” and colour it white instead of yellow. Then under F♯ there would be a blue column of F starting in the second row and going all the way down, giving two blue staircases of flatted notes as you move through the modes. Much more pleasing (even more so if you renamed F♯ to F and F to F♭).
If that went by too fast let me explain it. First, he's saying that the anomalous yellow F♯ in this chart comes from us taking major, or the Ionian mode, as our default mode:
Compared to Ionian, Lydian is the only mode that has a sharper note, shown in yellow. All the rest have flatter notes, shown in blue.
But suppose we bow to George Russell's logic and take Lydian as our default mode. Then because Lydian is the brightest mode, all other modes have only flatter notes, as shown in blue here:
And look! Now the pattern of flatting notes is much more systematic and beautiful!
I'll explain this pattern in a lot more detail next time, but first let's think about another thing Mark Reid just said. The fact that we call the special note in Lydian F♯ is not a law of nature. It's an artifact of our convention of taking major as the default mode!
Since we take major as our default, we call the notes in the C major scale C, D, E, F, G, A, and B. But if we took Lydian as our default we'd call the notes in C Lydian C, D, E, F, G, A, and B. In other words, what we now call F♯ we would call F. So what we now call F, we would call F♭.
And if we used this Lydian-centered approach to naming notes, our chart of modes would look even more logical:
Each time we go down to a darker mode, we just add a flat symbol to one more note! Wonderful!
But as Toby Bartels pointed out on my blog, we don't have to mess with our usual convention of naming notes if we start our modes on F rather than C. Then we get this chart:
Next time I'll discuss the other thing Mark Reid told me. That's when everything really fell into place. But maybe you can figure it out yourself. I've just told you two facts about the Lydian mode. First, if you start with one note and ascend the circle of fifths until you get seven notes, these are the notes in the Lydian mode:
Second, the Lydian mode is the brightest of the seven modes of the major scale. You can get all the rest by flatting one note after another following this pattern:
How are these two facts connected?
August 10, 2023
If you start at any note of the piano and keep going up fifths until you've got a total of 7 notes, you get the Lydian mode. I explained this last time.
Above I show how it works starting with the note C. If we take these 7 notes and list them in increasing order starting with C, we get
which is C Lydian. Great! But please remember that C is an arbitrary choice of starting note. If we'd started at any other note we'd still get a Lydian scale.
Lydian is mostly made from notes of the major scale — with one exception, which shows up as the F♯ in the above example. What if we want the major scale, also called the Ionian mode? Then we should go up by fifths until we get 6 notes, but also go down a fifth to get one more:
C is still in red, which means the scale starts there! If we start there and write the 7 notes of the scale in increasing order, we get
which is C Ionian. Again, there's nothing special about C here. The whole story I'm telling would work just as well with any other note.
It's fun to keep playing this game. Let's start at C and go up by fifths until we get 5 notes, but also go down by fifths to get 2 more:
Now we get C Mixolydian:
Next let's start at C and go up by fifths until we get 4 notes, but also go down by fifths to get 3 more
Now we get C Dorian:
Next let's start at C and go up by fifths until we get 3 notes, but also go down by fifths to get 4 more:
Now we get C Aeolian, also called C natural minor:
Next let's start at C and go up by fifths until we get 2 notes, but also go down by fifths to get 5 more:
Now we get C Phrygian:
Finally we can start at C and just go down by fifths to get 6 more notes:
Now we get C Locrian:
Now we've created all 7 modes of the major scale starting at C. If we list them in the order they were created, we get this chart:
We can see some interesting things here. As we work our way down the chart, each new mode differs from the previous one by having one of its notes lowered a half-step! I show this by having the note turn blue. We say each mode is 'darker' than the previous one.
There's an interesting pattern in how the notes get lowered. Let's understand it! Here's the order in which notes get lowered as we move down the chart:
Each of these notes is a fifth below the one before!
It's easy to see why this happens from all the pictures I drew. In each new mode we added a new note that's a fifth below the last one we added. We can see them all in the very last picture, showing the darkest of our modes:
See? Working down from C, which is the note that appears in all 7 modes we're discussing, we get
as we go around, each note being a fifth below the previous one!
I hope that's clear. Now for another question: what if we extend this chart by lowering the one tone that hasn't been lowered yet, the C?
We get another mode of the major scale: C♭ Lydian!
And the pattern of blue bars is beautifully continued! That's because C♭ is a fifth below the previous lowered note, G♭.
It's really cool how by lowering the one tone that hadn't been lowered — the root of the scale, the so-called 'tonic' — we suddenly pop from the darkest mode, Locrian, back to the brightest mode, Lydian. Why is that? It's easiest to see using our diagrams. Let's compare C Locrian to C♭ Lydian:
In terms of the notes these scales contain, the only difference is that we've replaced C by C♭. But this lowers the very bottom note of the scale. And this means that instead of the notes being bunched up near the bottom of the scale, making the scale very dark, they are now bunched up near the top, making it very bright. By lowering the bottom note, the other notes become higher by comparison!
Now, you may have been wanting to complain that C♭ isn't a thing:
You would be wrong. C♭ really does exist: in various contexts, like listing the notes in a scale one letter at a time, musicians do have good reasons to call one of those notes C♭. This is actually a rather deep topic:
But in my series on modes I'm only talking about the tones an ordinary modern piano can play, tuned in equal temperament, so some of the nuances Adam Neely discusses are not relevant here. For our discussion now, C♭ is just another name for B.
In short, while C♭ Lydian does exist, when played on a modern piano it sounds just like B Lydian. So let's redraw our chart, calling it B Lydian:
A bunch of notes in the last row now get new names, with sharps rather than flats, to make sure that each letter from A to G appears once. But they still sound the same.
More importantly: now we are back to Lydian, so we can play the game all over again!
We can lower one tone at a time, just as we did before, and go through the 7 modes from B Lydian to B Locrian. And then we can lower that B a half-tone, and so on:
This chart could go on forever! In each row we lower one note by a half-step. It's always a fifth below the note we lowered in the previous row. The chart eventually repeats. But it repeats only after we've covered all 7 modes of the major scale starting on all 12 notes in the chromatic scale — a total of 84 modes!
(If you have the ability to create a beautiful long chart like this without dying of boredom, please send it to me.)
It's fun to think about exactly why when we lower the first note in C Locrian we get B Lydian — why the darkest mode suddenly transforms into the brightest one. I think I'll let you ponder that.
All the ideas in this post emerged from a short comment by Mark Reid on Mastodon:
Furthermore, which note is flatted cycles through the circle of fifths. If you continue the pattern past the bottom row the next note to be flattened would be the C down to a B, creating a B Lydian scale. So the whole pattern through the modes repeats a half step down.
I am apparently much less efficient at transmitting information! But I had to imagine those wheel-shaped charts to really appreciate why things work as they do. And it may pay to ponder those charts, since they reveal a lot of interesting patterns.
August 11, 2023
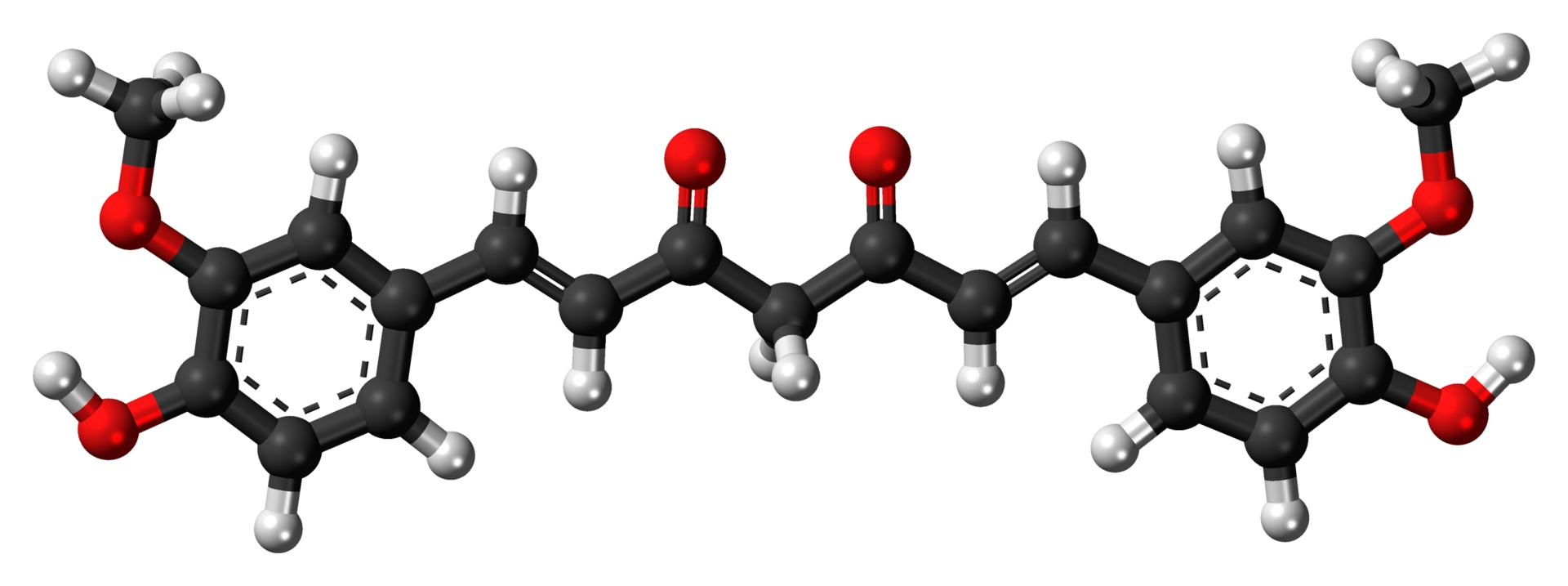
Suppose you were trying to invent a bright orange powder that could easily dye clothes and be hard to wash off. Using your knowledge of quantum mechanics you'd design this symmetrical molecule where an electron's wavefunction can vibrate back and forth along a chain of carbons at the frequency of green light. Absorbing green light makes it look orange! And this molecule doesn't dissolve in water.
Yes: you'd invent turmeric!
Or more precisely 'curcurmin', the molecule that gives turmeric its special properties. The black atoms are carbons, the white are hydrogens and the red are oxygens.
Ain't it pretty?

People extract curcumin from turmeric to use as a food coloring in curry powders, mustards, butters, cheeses, and prepared foods. It's also used in dietary supplements due to its unproven and dubious health benefits.
It doesn't dissolve well in water, but it does in alcohol. If you dissolve some curcurmin in vodka and shine a black light on it, you'll see it's fluorescent! That is: it absorbs the high-energy ultraviolet photons and emits lower-energy green photons... the same kind of light it usually likes to absorb. Due to the principle of reciprocity, if a substance is good at absorbing some frequency of light, it's also good at emitting that frequency.
Here's curcumin dissolved in a hydrocarbon called xylene with ultraviolet light shining on it!

It's fluorescent. You can also dissolve it in ethanol, e.g. vodka.
Curcurmin also makes a good pH detector: if you mix it with a base it
turns red. This video by Compound Interest illustrates it:
On August 7th and August 10th we used George Russell's theories about the Lydian mode to illuminate some beautiful patterns in the modes of the major scale.
Now let's make those patterns more precise using a little group theory. We'll see that the 84-element group
acts as symmetries on the set of all modes of the major scale, in all keys! This group combines the symmetries of the 12-tone scale of black and white keys on the piano (the chromatic scale) and the 7-tone scale of just white keys (the diatonic scale).
Before diving in, let's recall what we discovered last time. We saw that starting from Lydian we can get all modes of the major scale by successively lowering notes by a half-tone:
When we lower a note, it turns blue in this chart. Staring at the pattern you can see that each note being lowered is a fifth above the previous note that was lowered — or a fourth below, but I want to emphasize fifths.
This is great, but it gets really interesting when we continue the pattern. The last note we lowered was F. If we go up a fifth from that we get C. Hey! This was the only note we haven't lowered yet! If we lower the C a half-tone we get B — and we get an extra row in our chart:
Now we're back to Lydian! But now it's B Lydian.
We can keep playing this game. In fact we can go on forever. We'll eventually loop back to C Lydian and repeat — but only after we've covered all 7 modes starting on all 12 notes in the chromatic scale! That's a total of 84 modes.
Last time I asked if someone could make a really long version of my chart listing all 84 modes. Wyrd Smythe rose to the challenge and produced this magnificent chart:
| Lydian | C | D | E | F♯ | G | A | B |
|---|---|---|---|---|---|---|---|
| Ionian | C | D | E | F | G | A | B |
| Mixolydian | C | D | E | F | G | A | B♭ |
| Dorian | C | D | E♭ | F | G | A | B♭ |
| Aeolian | C | D | E♭ | F | G | A♭ | B♭ |
| Phrygian | C | D♭ | E♭ | F | G | A♭ | B♭ |
| Locrian | C | D♭ | E♭ | F | G♭ | A♭ | B♭ |
| Lydian | B | C♯ | D♯ | E♯ | F♯ | G♯ | A♯ |
| Ionian | B | C♯ | D♯ | E | F♯ | G♯ | A♯ |
| Mixolydian | B | C♯ | D♯ | E | F♯ | G♯ | A |
| Dorian | B | C♯ | D | E | F♯ | G♯ | A |
| Aeolian | B | C♯ | D | E | F♯ | G | A |
| Phrygian | B | C | D | E | F♯ | G | A |
| Locrian | B | C | D | E | F | G | A |
| Lydian | B♭ | C | D | E | F | G | A |
| Ionian | B♭ | C | D | E♭ | F | G | A |
| Mixolydian | B♭ | C | D | E♭ | F | G | A♭ |
| Dorian | B♭ | C | D♭ | E♭ | F | G | A♭ |
| Aeolian | B♭ | C | D♭ | E♭ | F | G♭ | A♭ |
| Phrygian | B♭ | C♭ | D♭ | E♭ | F | G♭ | A♭ |
| Locrian | B♭ | C♭ | D♭ | E♭ | F♭ | G♭ | A♭ |
| Lydian | A | B | C♯ | D♯ | E | F♯ | G♯ |
| Ionian | A | B | C♯ | D | E | F♯ | G♯ |
| Mixolydian | A | B | C♯ | D | E | F♯ | G |
| Dorian | A | B | C | D | E | F♯ | G |
| Aeolian | A | B | C | D | E | F | G |
| Phrygian | A | B♭ | C | D | E | F | G |
| Locrian | A | B♭ | C | D | E♭ | F | G |
| Lydian | G♯ | A♯ | B♯ | C♯♯ | D♯ | E♯ | F♯♯ |
| Ionian | G♯ | A♯ | B♯ | C♯ | D♯ | E♯ | F♯♯ |
| Mixolydian | G♯ | A♯ | B♯ | C♯ | D♯ | E♯ | F♯ |
| Dorian | G♯ | A♯ | B | C♯ | D♯ | E♯ | F♯ |
| Aeolian | G♯ | A♯ | B | C♯ | D♯ | E | F♯ |
| Phrygian | G♯ | A | B | C♯ | D♯ | E | F♯ |
| Locrian | G♯ | A | B | C♯ | D | E | F♯ |
| Lydian | G | A | B | C♯ | D | E | F♯ |
| Ionian | G | A | B | C | D | E | F♯ |
| Mixolydian | G | A | B | C | D | E | F |
| Dorian | G | A | B♭ | C | D | E | F |
| Aeolian | G | A | B♭ | C | D | E♭ | F |
| Phrygian | G | A♭ | B♭ | C | D | E♭ | F |
| Locrian | G | A♭ | B♭ | C | D♭ | E♭ | F |
| Lydian | F♯ | G♯ | A♯ | B♯ | C♯ | D♯ | E♯ |
| Ionian | F♯ | G♯ | A♯ | B | C♯ | D♯ | E♯ |
| Mixolydian | F♯ | G♯ | A♯ | B | C♯ | D♯ | E |
| Dorian | F♯ | G♯ | A | B | C♯ | D♯ | E |
| Aeolian | F♯ | G♯ | A | B | C♯ | D | E |
| Phrygian | F♯ | G | A | B | C♯ | D | E |
| Locrian | F♯ | G | A | B | C | D | E |
| Lydian | F | G | A | B | C | D | E |
| Ionian | F | G | A | B♭ | C | D | E |
| Mixolydian | F | G | A | B♭ | C | D | E♭ |
| Dorian | F | G | A♭ | B♭ | C | D | E♭ |
| Aeolian | F | G | A♭ | B♭ | C | D♭ | E♭ |
| Phrygian | F | G♭ | A♭ | B♭ | C | D♭ | E♭ |
| Locrian | F | G♭ | A♭ | B♭ | C♭ | D♭ | E♭ |
| Lydian | E | F♯ | G♯ | A♯ | B | C♯ | D♯ |
| Ionian | E | F♯ | G♯ | A | B | C♯ | D♯ |
| Mixolydian | E | F♯ | G♯ | A | B | C♯ | D |
| Dorian | E | F♯ | G | A | B | C♯ | D |
| Aeolian | E | F♯ | G | A | B | C | D |
| Phrygian | E | F | G | A | B | C | D |
| Locrian | E | F | G | A | B♭ | C | D |
| Lydian | E♭ | F | G | A | B♭ | C | D |
| Ionian | E♭ | F | G | A♭ | B♭ | C | D |
| Mixolydian | E♭ | F | G | A♭ | B♭ | C | D♭ |
| Dorian | E♭ | F | G♭ | A♭ | B♭ | C | D♭ |
| Aeolian | E♭ | F | G♭ | A♭ | B♭ | C♭ | D♭ |
| Phrygian | E♭ | F♭ | G♭ | A♭ | B♭ | C♭ | D♭ |
| Locrian | E♭ | F♭ | G♭ | A♭ | B♭♭ | C♭ | D♭ |
| Lydian | D | E | F♯ | G♯ | A | B | C♯ |
| Ionian | D | E | F♯ | G | A | B | C♯ |
| Mixolydian | D | E | F♯ | G | A | B | C |
| Dorian | D | E | F | G | A | B | C |
| Aeolian | D | E | F | G | A | B♭ | C |
| Phrygian | D | E♭ | F | G | A | B♭ | C |
| Locrian | D | E♭ | F | G | A♭ | B♭ | C |
| Lydian | C♯ | D♯ | E♯ | F♯♯ | G♯ | A♯ | B♯ |
| Ionian | C♯ | D♯ | E♯ | F♯ | G♯ | A♯ | B♯ |
| Mixolydian | C♯ | D♯ | E♯ | F♯ | G♯ | A♯ | B |
| Dorian | C♯ | D♯ | E | F♯ | G♯ | A♯ | B |
| Aeolian | C♯ | D♯ | E | F♯ | G♯ | A | B |
| Phrygian | C♯ | D | E | F♯ | G♯ | A | B |
| Locrian | C♯ | D | E | F♯ | G | A | B |
My former master's student Owen Lynch made a chart that literally loops around:
The only problem with this chart is that you have to make it really big to see it. Click on it and enlarge it as much as you can! Or check out the source code on GitHub.
(The really tricky part about both these charts is that some modes require notes like B double flat, sometimes written B𝄫, or C double sharp, sometimes written C𝄪. These notes are enharmonically equivalent to other notes with simpler names: for example B𝄫 sounds just like A on a standard modern keyboard. But musicians demand that each of the seven letters ABCDEFG shows up exactly once in each mode, which is actually quite reasonable. When it comes to the math, this purely notational issue is just a sideshow.)
Now, when I pondered this loop of 84 modes, it made me think of the cyclic group \(\mathbb{Z}/84.\) And then I realized what was going on.
The notes of the chromatic scale form the group \(\mathbb{Z}/12.\) So, each of the 84 modes determines a 7-element subset of \(\mathbb{Z}/12.\)
But we only get certain special 7-element subsets this way. As we saw last time, these are the subsets where we start anywhere and keep going up by fifths until we have 7 elements, like this:
A fifth equals 7 half-tones, so these subsets are of all this form:
where of course we're doing addition mod 12. We can also say it this way:
This is cuter because it has two sevens in it! This reflects a curious coincidence: there are 7 notes in the major scale, or any mode of the major scale, but also the interval of a fifth is 7 half-tones.
So far so good. But our 84 modes are more than mere subsets: each mode also has a chosen 'tonic’, or starting point! For example, C Lydian has C as the tonic, drawn in red:
But G Ionian is the same set of notes with G as the tonic:
So for us, right now, a mode will be a set of the form \(S(n) \subset \mathbb{Z}/12\) together with a chosen element of this set, which we call the tonic.
Once we think of things this way, we can see how the group \(\mathbb{Z}/12 \times \mathbb{Z}/7\) acts on the set of modes. It acts because we have an action of \(\mathbb{Z}/12\) and an action of \(\mathbb{Z}/7,\) and these commute with each other.
The group \(\mathbb{Z}/12\) acts on modes an obvious way: we just raise or lower every note in the set \(S(n),\) along with the tonic, by the same amount. Musicians call this 'transposition’.
The group \(\mathbb{Z}/7\) acts by cycling the tonic around within the set \(S(n).\) For example if we start with C Ionian and cycle the tonic around we get these 7 modes:
and then we loop around back to C Ionian. Here I'm showing the tonic in boldface.
Now for a cool mathematical fact:
$$ \mathbb{Z}/12 \times \mathbb{Z}/7 \cong \mathbb{Z}/84 $$
That's because 7 and 12 are relatively prime. And, even better, there's an element of \(\mathbb{Z}/84\) that generates this group, which has a magical property: starting with any mode, and repeatedly acting by this element, we loop around all 84 modes!
What is this magical element? It's
$$ (-7,4) \in \mathbb{Z}/12 \times \mathbb{Z}/7 $$
That is, first we transpose all the notes in our mode down by 7 half-tones, as well as the tonic. Then we cycle the tonic 4 steps upward within the mode.
The point here is that cycling the tonic 4 steps upward within the mode usually moves it up 7 half-tones, but in one case just 6. So usually the combined process leaves the tonic alone, but in one exceptional case it moves down by a half-tone! And that generates this process:
The one exceptional case is when we move from Locrian to Lydian. That's when the tonic moves down a half-tone!
Let's see how this works in a couple examples. But first I want to re-emphasize that this 'glitch':
is not something new. We saw it last time. 7 half-tones is a fifth, and it's not always true that going up a fifth stays within the same mode:
In our friend C Ionian, shown above, the glitch happens at B. If we go up a fifth from B we 'fall off the edge' of C Ionian and get F♯. But this glitch happens in every mode. And this glitch is exactly what connects all 84 modes of all scales into a single loop!
Now let's look at two examples.
First try the Lydian mode. To be specific, C Lydian:
Transpose all the notes and the tonic down by 7 half-tones:
Then cycle the tonic upward 4 steps within the mode:
This is C Ionian! Note that in our two-step process we first lowered the tonic by 7 half-tones from C to F, and then raised it by 7 half-tones from F back to C. So the tonic remained unchanged.
That's how it works for most modes. But for one it works differently! And that's Locrian: the ugly duckling of the modes.
For example, let's look at C Locrian:
Transpose all the notes and the tonic down by 7 half-tones:
Then cycle the tonic upward 4 steps within the mode:
This is B Lydian! Note we first lowered the tonic by 7 half-tones, from C to E♯. Then we raised it by just 6 half-tones, from E♯ to B. So this time the tonic went down a half-tone.
To conclude, let me say a lot of this again, but more mathematically and much faster. To describe a mode we choose its set of notes
$$ S(n) = \{n + 7i \; \vert \; 1 \le i \le 7 \} \subset \mathbb{Z}/12 $$
and then choose the tonic \(t \in S(n).\) All this information is captured by a function
$$ f \colon \mathbb{Z}/7 \to \mathbb{Z}/12 $$
that lists the elements of \(S(n)\) in cyclic order, starting with the tonic: \(f(0) = t.\)
Now for the cool part. Since the function \(f\) captures all the information in the mode, we can say a mode is a function
$$ f \colon \mathbb{Z}/7 \to \mathbb{Z}/12 $$
of the above sort. Then the action of \(\mathbb{Z}/12 \times \mathbb{Z}/7\) on the set of modes is just what any healthy, alert mathematician would guess! \(\mathbb{Z}/7\) acts on the domain and \(\mathbb{Z}/12\) acts on the codomain. That is,
for any \((n,i) \in \mathbb{Z}/12 \times \mathbb{Z}/7\) and \(j \in \mathbb{Z}/7.\)
And the magical element \((-7,4),\) which generates the group \(\mathbb{Z}/12 \times \mathbb{Z}/7,\) acts to move us around the grand loop of 84 modes!
Finally, for the real math whizzes out there: since the group \(\mathbb{Z}/12 \times \mathbb{Z}/7\) acts freely and transitively on the set of 84 modes, which is nonempty, we say the set of modes is a torsor of that group. Elsewhere I've explained torsors:
and explained how the set of major and minor triads forms a torsor for the 24-element dihedral group in two different ways:
So it is nice to see torsors showing up yet again in music. And we could fit the 24-element dihedral group and today's 84-element group inside a 168-element group that includes today's 84-element group and also inversions... but I'm getting tired, and I imagine you are too.
You can see my discussions of modes on my blog here:
© 2023 John Baez
baez@math.removethis.ucr.andthis.edu