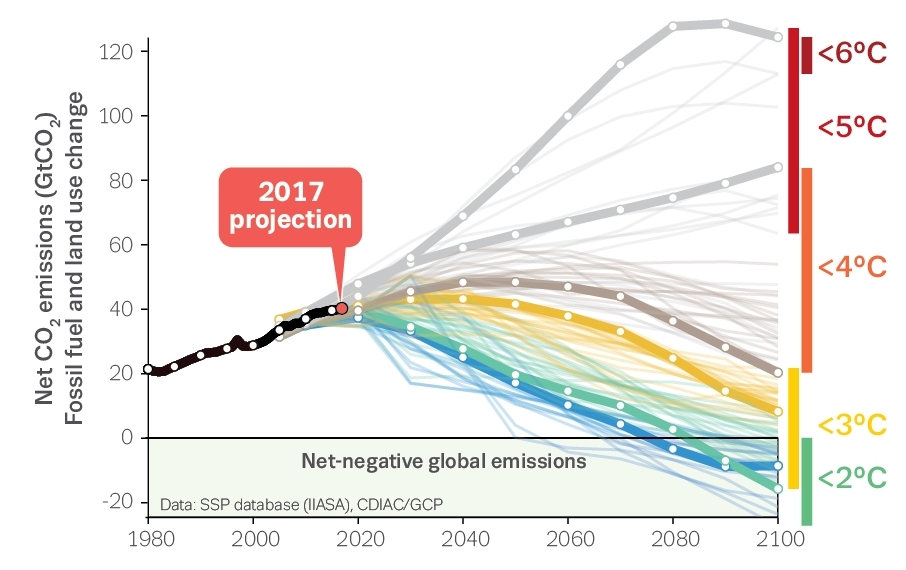
The weather disasters we're seeing will keep getting worse unless we cut carbon emissions until they're negative. That's hard. But how much is the Paris Agreement helping?
The EU committed to cut carbon emissions by 40% from 1990 to 2030. They'd gone down 23% by 2016 — but last year they went up. The EU's energy chief wants to do more: cut by 45%. But Merkel is pushing back. Germany is already going to miss its 2020 target.
The US pledged to cut carbon emissions 17% from 2005 to 2020, and 26% by 2025. Trump pulled us out of the agreement. Many in the US are soldiering on, but right now it looks like we'll miss these goals.
China's carbon emissions shot up starting in 2000. It leveled off after 2010, even going down a bit — but it went up 3.5% last year. India and "ROW" (rest of world) are also going up.
China pledged only to reach peak carbon emissions by 2030. See what other countries pledged — and how they're doing:
Short version: right now we're heading for 2.5-4.7°C warming by 2100. Between bad and disaster.
But some good news...
California just passed a law saying we'll switch to completely carbon-free electricity production by 2045! Very hard, but look at this chart:
California's carbon emissions per GDP has dropped almost 50% from 2000
to 2016! And today Governor Brown announced: "with climate science
still under attack... we'll launch our own damn satellite" to track
carbon emissions.
September 14, 2018

Lisa and I have been in Singapore since August 10th, and we're leaving soon. As usual, I've been visiting the Centre for Quantum Technologies. Today I gave a talk on Noether's theorem relating symmetries and conserved quantities. It's the 100th anniversary of her paper on this! But we still haven't gotten to the bottom of it.
Noether showed that in a theory of physics obeying the principle of least action, any 1-parameter family of transformations preserving the action gives a conserved quantity. But Noether's theorem takes different guises in other approaches to physics, and my talk focuses on the algebraic approach using Poisson brackets or commutators. I argue that this explains the role of complex numbers in quantum theory!
I'll also give this talk at the Department of Applied Mathematics and Theoretical Physics (DAMTP) at Cambridge, and in a conference celebrating the anniversary of Noether's theorem in London. You can see my slides here:
Abstract. In her paper of 1918, Noether's theorem relating symmetries and conserved quantities was formulated in term of Lagrangian mechanics. But if we want to make the essence of this relation seem as self-evident as possible, we can turn to a formulation in term of Poisson brackets, which generalizes easily to quantum mechanics using commutators. This approach also gives a version of Noether's theorem for Markov processes. The key question then becomes: when, and why, do observables generate one-parameter groups of transformations? This question sheds light on why complex numbers show up in quantum mechanics.
The quaternion group has \(8\) elements. Two of them, \(\pm 1\), commute with everything. The rest commute with \(4\) elements each: for example \(i\) commutes with \( \pm 1, \pm i\).
So, one quarter of the elements commute with every element. Three quarters of the elements commute with half. Thus the probability that two randomly chosen elements in this group commute is $$ \frac{1}{4} \times 1 + \frac{3}{4} \times \frac{1}{2} = \frac{5}{8} $$ But what's the largest this probability can be, for any noncommutative group?
It's 5/8. And I explain why here:

But what the hell are Sylow's theorems good for? As an undergrad in love with physics, I never really got it. Now on YouTube you can see examples that show what you can do with them — nice!
You can show there are no simple groups of order 30:
You can show that any group of order 77 is cyclic:
Okay, but how do you prove them?
You can also see proofs of Sylow's theorems on YouTube, like this, the first of a 3-part series.
But I'm often too impatient for videos. I'd rather see really short proofs, then think about them in my spare time: washing the dishes, lying in bed, etc.
So here's a really short proof of all 3 Sylow theorems from Robert A. Wilson's book Finite Simple Groups. This proof takes lots of work to unravel. But I love it: all the ideas are here.
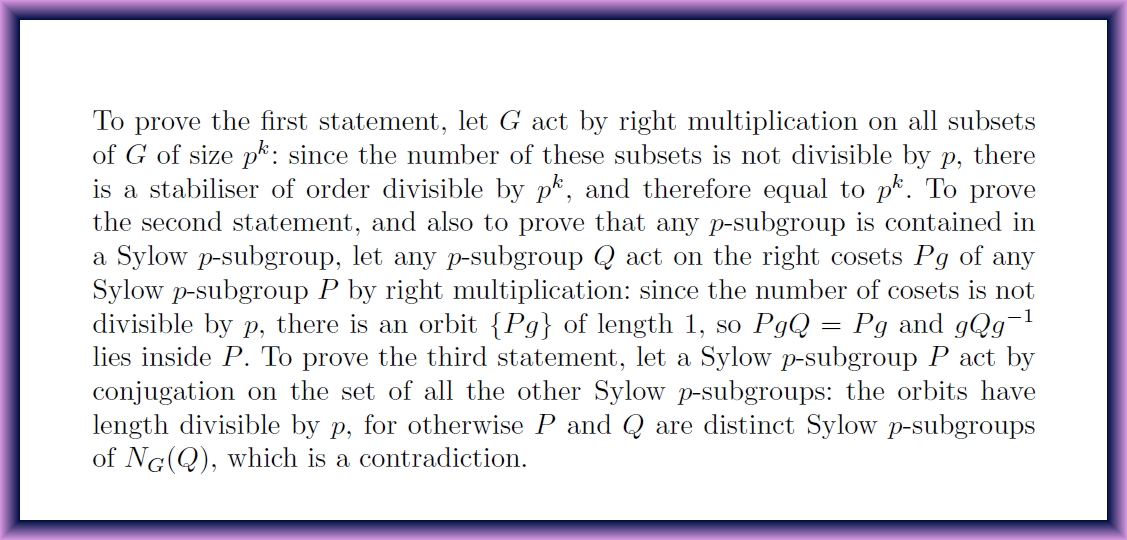
Here \(N_G(Q)\) is the normalizer of \(Q\) in \(G\).
September 20, 2018
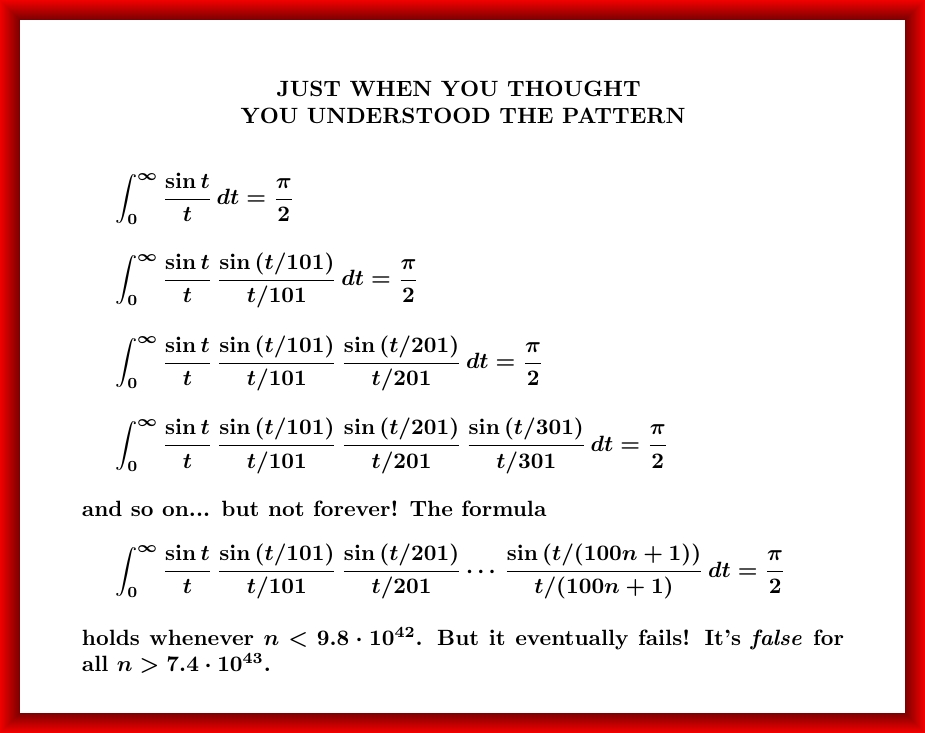
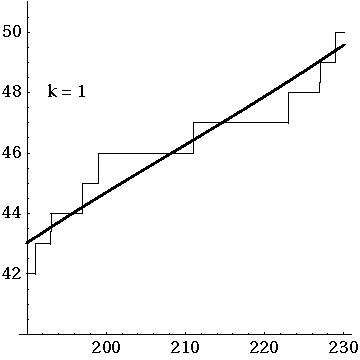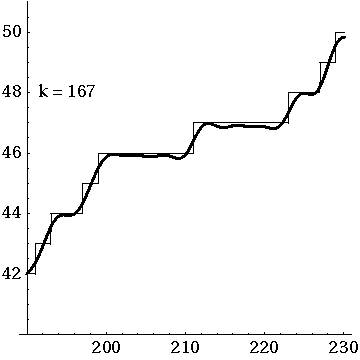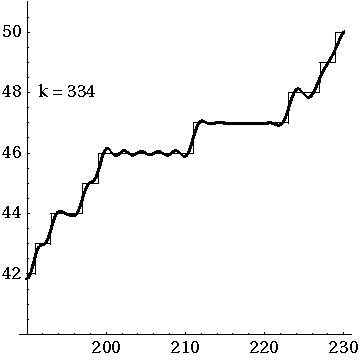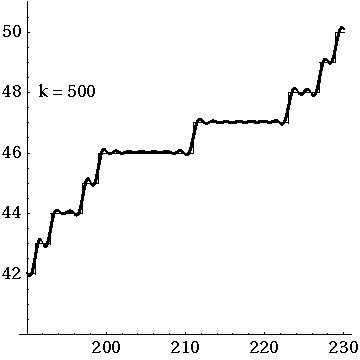
Sometimes you check just a few examples and decide something is always true. But sometimes even \(9.8 \times 10^{42}\) examples are not enough!
Greg Egan and I came up with this shocker on Twitter after he explained some related integrals by the Borwein brothers. To see what's really going on, visit my blog:
The Riemann zeta function is given by this simple formula when the complex number \(s\) has \(\mathrm{Re}(s) \gt 1\): $$ \zeta(s) = \sum_{n = 1}^\infty \frac{1}{n^s} $$ Then the sum converges! But we can "analytically continue" the Riemann zeta function to define it for other values of \(s\), and that's where the fun starts.
The Riemann zeta function is zero for some numbers with \(0 \lt \mathrm{Re}(s) \lt 1\). These are called the "nontrivial zeros" of his zeta function. Riemann computed a few and hypothesized they all have \(\mathrm{Re}(s) = 1/2\).
Riemann found a formula for the number of primes \(\lt n\) as a sum over the nontrivial zeros of the zeta function. My first tweet shows the sum over the first k nontrivial zeros. So, if the Riemann Hypothesis is true, we'll get a better understanding of primes!
For example, in 2014, Adrian Dudek proved that the Riemann hypothesis implies that that for all \(n \ge 2\) there is a prime \(p\) with $$ n - \frac{4}{\pi} \sqrt{x} \ln x \lt p \le n $$ So far people have checked, using a computer, that the first 10,000,000,000,000 nontrivial zeros of the Riemann zeta function have \(Re(s) = 1/2\). This might seem like damned good evidence for the Riemann Hypothesis. But maybe not!

For me, the best evidence for the Riemann Hypothesis is that it's part of a much bigger story! Mathematicians like Weil, Grothendieck and Deligne proved similar results for related functions. Much remains mysterious, though.
I bet that Atiyah's claimed proof, if and when he writes it up, will not convince experts. In 2017 he claimed to have a 12-page proof of the Feit-Thompson theorem, which usually takes 255 pages:
In 2016 Atiyah put a paper on the arXiv claiming to have solved a famous problem in differential geometry. The argument was full of big holes:
So, I'm not holding my breath this time.
Here, apparently, is his paper:
It refers extensively to this much longer paper, where he attempts to compute the fine structure constant:
Here's what Science says about Atiyah:
Only a few mathematicians were willing to be quoted, and I was one. I was also quoted later here:
By the way, I have huge respect for Atiyah, whose earlier work
revolutionized geometry and physics.
September 29, 2018
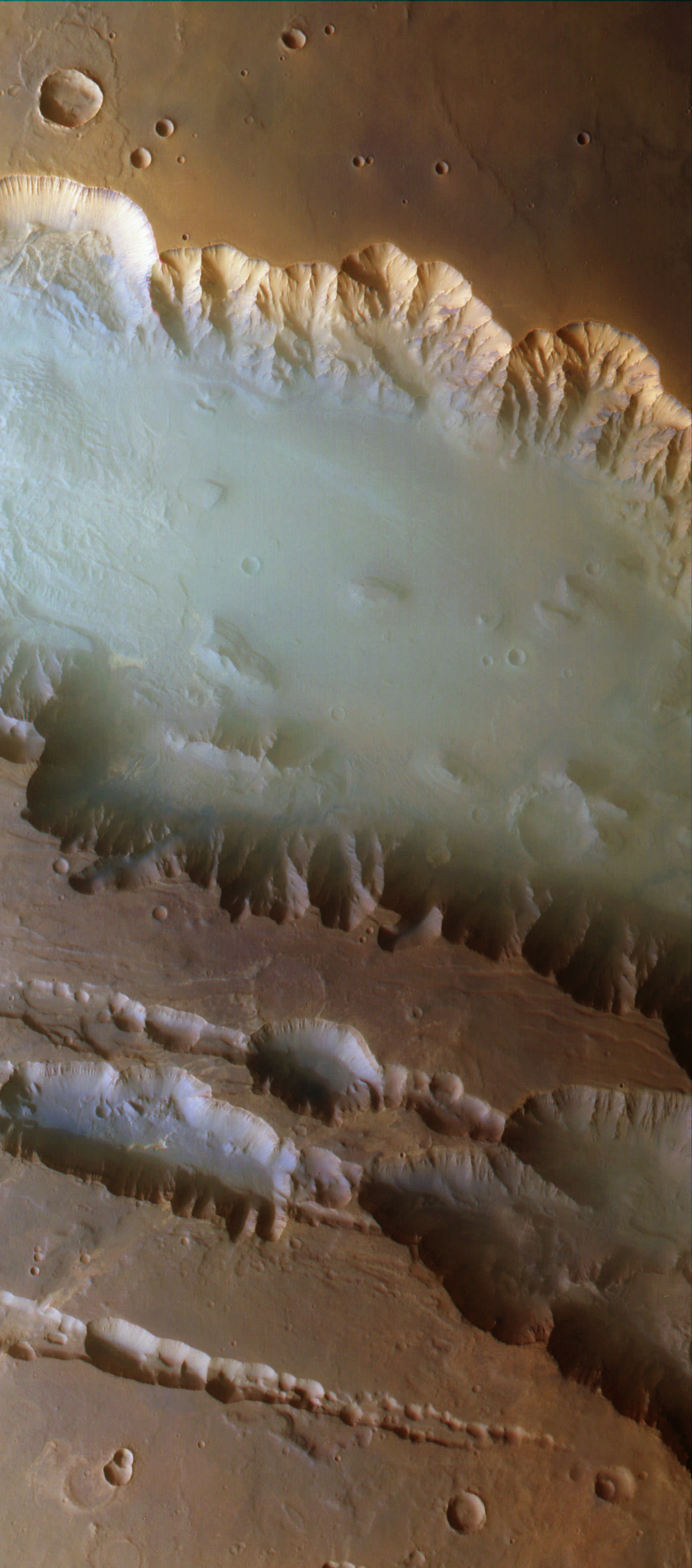
Fog in a Martian canyon on a chilly morning! This is a high-resolution image of Valles Marineris: a huge canyon on Mars, 4000 kilometers long and up to 7 kilometers deep.
Here's another view:

This fog is made of water ice, not liquid water, so it's a bit like a Martian relative of 'pogonip': a dense winter frozen fog in mountain valleys. But it's different: those mountain valleys are colder than the uplands, but Valles Marineris is warmer than the higher regions nearby! This may indicate that there's source of water in the valley, that makes it more humid. It could be frost submlimated by the morning sun.
And here's a view of fog in Noctis Labyrinthus — a maze-like system of deep, steep-walled valleys between Valles Marineris and the nearby Tharsis upland.
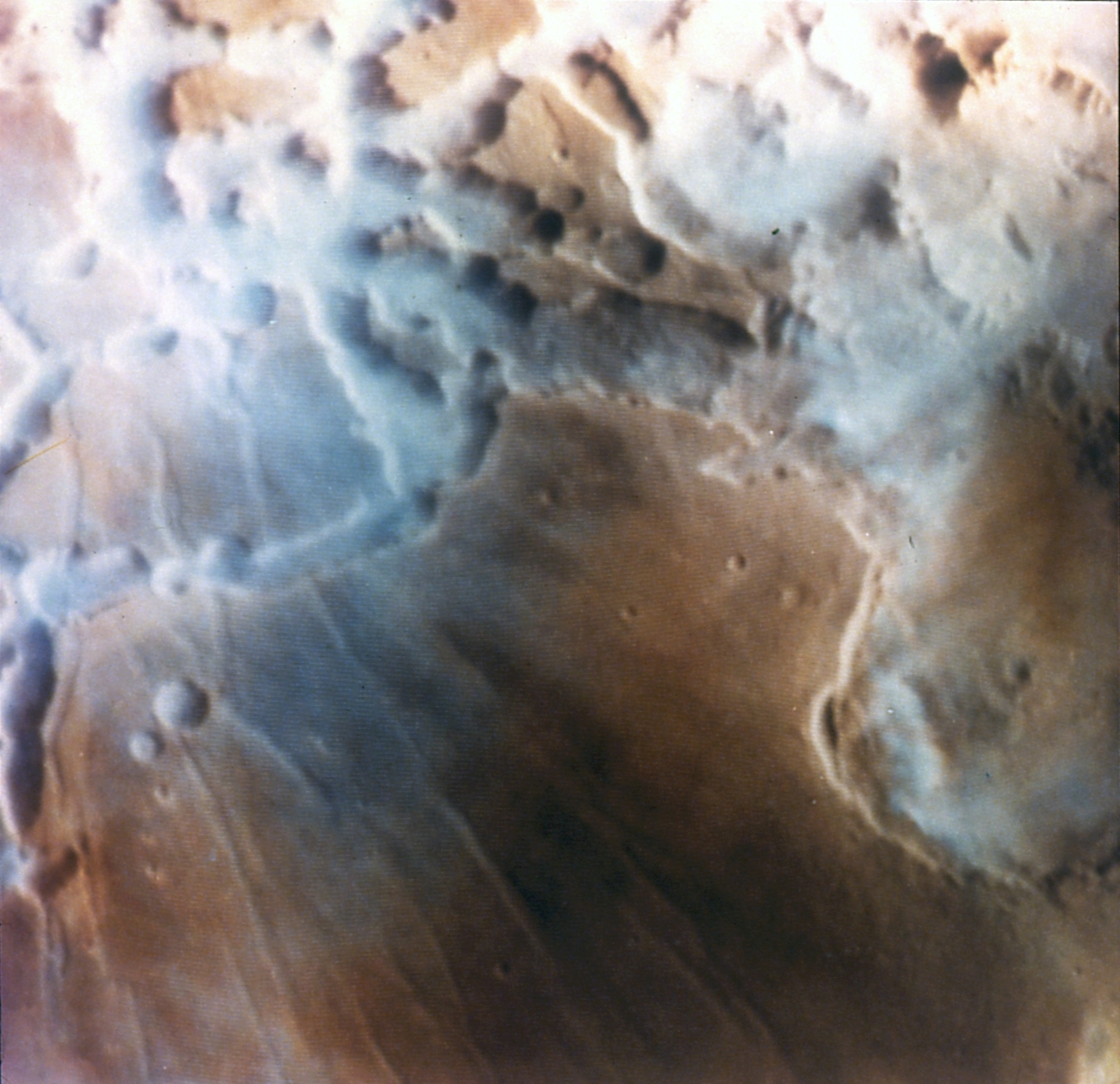
Fog in the Labyrinth of the Night. How poetic! I would like to see this someday.