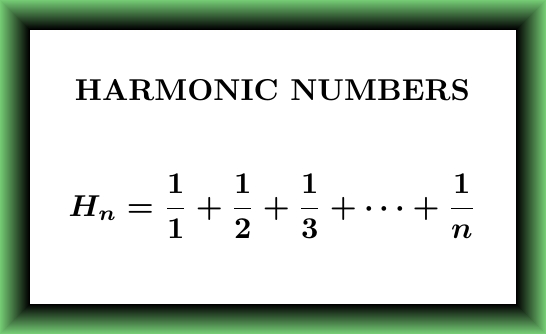
Harmonic numbers are numbers like \(1 + 1/2 + 1/3 + 1/4\). They're pretty interesting!
The harmonic numbers grow step by step but they get close to a very nice smooth function. This function is a little bit more than the natural logarithm. "A little bit" is Euler's constant $$ \begin{array}{ccl} \gamma &=& \displaystyle{ \lim_{n \to \infty} \left( \sum_{i = 1}^n \frac{1}{i} - \int_1^n \frac{dx}{x} \right)} \\ \\ &=& 0.5772156649015328606\dots \end{array} $$ If you can prove this is irrational, you'll be famous!
Euler came up with a nice formula for the harmonic series too. It's easy to prove this using the geometric series.
The moral: do easy things before other people think of them.
This formula lets you define the harmonic number \(H_n\) even when \(n\) is
not an integer!
July 2, 2019
This is a really juicy result on random permutations!
I knew about the case \(k = 1\). If you randomly choose one of the \(n!\) permutations of an \(n\)-element set, the chance that it has no fixed points approaches \(1/e\) when \(n\) approaches infinity. But this is even better.
I found this result here:
Euler suspected that \(e^\gamma\) would be interesting and this is some evidence that he was right.
The "Ramanujan machine" has found some nice new continued fraction formulas! Are they really new? Are they really true? If so, how long will it take to prove them?
Here are some of the formulas discovered by this algorithm. It'll be fun to see what experts on Ramanujan-type continued fractions will say.
Are these easy consequences of known results, or will proving them require new ideas?
Here is the paper:

The famous set theorist Hugh Woodin recently gave some talks at the
National University of Singapore. The ad for his talks looks
amazing! I guess "living large" takes on a whole new meaning when you
study large cardinals.
July 25, 2019
The pentagram is packed with math. It's no surprise the Pythagoreans revered it. For starters, it has line segments of 4 different lengths, each related to the next by the golden ratio!
On top of that, if you draw a pentagram inside a pentagon you can see it contains 20 "golden triangles". These are isosceles triangles whose sides are related by the golden ratio.
It's funny how few people notice this. But to see, you have to look!
For more see:
Why are Penrose tilings so fascinating? One reason is that no pattern with 5-fold symmetry in the plane can repeat in a periodic way.
But why is this? It's because no lattice in the plane can contain a regular pentagon.
And why is that?
On Twitter, Joel David Hamkins presented a nice proof that no square lattice can contain a regular pentagon. It's a proof by contradiction.
If the points of a square lattice form a regular pentagon you can find a smallest possible pentagon of this sort. Rotate its edges 90° as shown above. Since the lattice is a square lattice, you get new edges going between lattice points... and they form a smaller regular pentagon!
But why can no lattice have vertices forming a regular pentagon? I posed that as a puzzle and Liu Yao came up with a very elegant proof.
First, recall that a lattice is a discrete set of vectors in the plane such that if two vectors are in the set, so are their sum and difference. Thus, if three vertices of a parallelogram are in a lattice, so is the fourth.
Now, suppose there is a regular pentagon whose vertices are the points of some lattice. There must be a smallest such pentagon — say, the big pentagon in this picture. The green dots form a parallelogram. Three are points in the lattice, so the fourth must be too! The same is true for all the vertices of the small pentagon in this picture. This gives a smaller regular pentagon whose vertices are in the lattice. Contradiction!
Like the proof by Joel David Hamkins, this is a proof by infinite descent: assume you have the smallest possible thing of some kind, and get a contradiction by finding an even smaller one. Fermat made such proofs famous, though there's an ancient Greek proof of the irrationality of \(\sqrt{2}\) that works the same way.
Because no lattice can contain a regular pentagon among its points, the symmetry group of such a pentagon can't act as symmetries of a lattice! We thus say this group is noncrystallographic.
On Twitter I challenged my readers to prove that the symmetry group of a regular heptagon is also noncrystallographic.
Joel David Hamkins pointed out that the exact same argument show that the symmetry of a regular n-gon is noncrystallographic except for n = 3, 4 or 6. Here's the idea:
He wrote:
It is interesting to ponder how the parallelogram argument breaks down for n = 3, n = 4 and n = 6. For n = 4, each parallelogram is the same as the original square. For n = 6, the new hexagon is degenerate, just one point. And for n = 3, the new points are outside the original triangle, and form a much larger triangle.
The result is far from new: people usually express it by saying the Coxeter
groups \(I_2(n)\) are noncrystallographic, hence not Weyl groups, for
n = 5, 7, 8, 9,.... This has many fascinating ramifications in
mathematics. But this proof is new to me, and appealingly visual.
July 29, 2019
The largest marsupial ever was the giant wombat, or "rhinoceros wombat". It lived in Australia. But what could eat a giant wombat — and when did they die out, and why?
One thing that might kill and eat a giant wombat was the giant monitor lizard Varanus priscus — another resident of Australia.
Both lived there until quite recently. They died out just 50,000 years ago, or even later! This is around when humans arrived in Australia. So, we may have killed them off, along with plenty of other megafauna around the world.
Sorry, giant wombat! We will never know you now.