_tiling.png)
_tiling.png)
What happened before the Big Bang? That's what I call a boring question.
It might not make sense. But don't believe anyone who confidently asserts that it doesn't make sense. It might make sense. We have no idea! We just don't know enough about physics to make much progress on this question right now! Maybe later.
What happened right after the Big Bang? That's much more interesting, because we don't know the complete answer, but we know a lot of stuff, and we have at least a chance of making progress.
Here's something easy you can do: take a solution of Einstein's equation for gravity, run it back in time, and see what it says about the shape of the universe as you get closer and closer to the Big Bang.
You might not think this is easy if you haven't taken a course on this stuff. But it's really easy compared to, say, building a telescope and sending it into orbit. You can do it with just a pencil and paper. So you might as well try it and see what you get.
In the simplest solutions, space is homogeneous and isotropic: for example completely flat, or completely round. Then it stays that way as you go back in time. That's what you usually read about in a basic course on this stuff.
But in some more interesting solutions, space is homogeneous but not isotropic. That means it looks the same at every location, but not the same in every direction.
In 1970, three Russian physicists named Belinskii, Khalatnikov and Lifshitz took these solutions, ran them back in time, and noticed something interesting. The universe oscillated in shape ever more wildly as time went back towards the Big Bang! And sometimes — depending on the particular solution — it would do so in a chaotic way.
Even better, they noticed that this problem was isomorphic to the problem of a ball rolling around in a 2-dimensional region.
"Isomorphic" means that the math works the same way after you change the names of things. For example, here, instead of working with time, you need to use minus the logarithm of time. As time goes to zero (back to the Big Bang), minus the logarithm of time keeps increasing forever. From this viewpoint there's time for a huge amount of happen as we get closer and closer to the Big Bang, but never quite get there!
And in these homogeneous but not isotropic solutions of the equations for gravity, as we get closer and closer to the Big Bang, the math works more and more like a billiard ball bouncing around in one of the triangles in this picture!
This picture shows the hyperbolic plane chopped up into triangles in a very symmetrical way. Pick any triangle; then a point in that triangle describes a possible shape of the universe in the solutions that Belinskii, Khalatnikov and Lifshitz were studying.
Of course their work is oversimplified, because it left out all the forces besides gravity, it ignored quantum mechanics, and it assumed the universe was homogeneous. So, don't take it too seriously! But still, it pointed out a new possibility: the universe could wiggle around more and more wildly as we run time back toward the Big Bang.
Even more importantly, from my perspective, it led to a huge amount of cool math connecting the equations of gravity to symmetrical ways of chopping up the hyperbolic plane into triangles, and higher-dimensional versions of that game. And that's what I really wanted to talk about today, but I see this post is getting too long, so I'll stop for now.
This is their paper:
Lifschitz, by the way, is one member of the famous physics textbook writing team Landau and Lifschitz. You can also learn more about the Belinksii-Khalatnikov-Lifschitz singularity here:

You know about crystals in space. What's a crystal in spacetime? It's a repetitive pattern that has a lot of symmetries including reflections, translations, rotations and Lorentz transformations. Rotations mix up directions in space. Lorentz transformations mix up space and time directions.
We can study spacetime crystals mathematically — and the nicest ones are described by gadgets called 'symmetrizable hyperbolic Dynkin diagrams', which play a fascinating role in string theory.
How do these diagrams work?
Each dot stands for a reflection symmetry of our spacetime crystal. Dots not connected by an edge are reflections along axes that are at right angles to each other. Dots connected by various differently labelled edges are reflections at various other angles to each other. To get a spacetime crystal, the diagram needs to obey some rules.
The number of dots in the diagram, called its 'rank', is the dimension of the spacetime the crystal lives in. So, the picture here shows a bunch of crystals in 5-dimensional spacetime.
Victor Kac, the famous mathematician who helped invent these spacetime crystals, showed they can only exist in dimensions 10 or below. He showed that:
In 1979, two well-known mathematicians named Lepowsky and Moody showed there were lots of spacetime crystals in 2 dimensions... but they classified all of them.
If they're right, there's a total of 142 spacetime crystals with dimensions between 3 and 10.
I think it's really cool how 10 is the maximum allowed dimension, and the number of spacetime crystals explodes as we go to lower dimensions.
String theory lives in 10d spacetime, so it's perhaps not very shocking that some 10-dimensional spacetime crystals are important in string theory — and also supergravity, the theory of gravity that pops out of superstring theory. The lower-dimensional ones seem to appear when you take 10d supergravity and 'curl up' some of the space dimensions to get theories of gravity in lower dimensions.
Greg Egan and I have been playing around with these spacetime crystals. I've spent years studying crystal-like patterns in space, so it's fun to start looking at them in spacetime. I'd like to say a lot more about them — but my wife is waiting for me to cook breakfast, so not now!
Nobody calls them 'spacetime crystals', by the way — to sound smart, you gotta say 'symmetrizable hyperbolic Dynkin diagrams'. Here's the paper by that big team:
By the way, there are also hyperbolic Dynkin diagrams that aren't symmetrizable, which don't give lattices. J Gregory Moxness created nice pictures of all 238 hyperbolic Dynkin diagrams with ranks between 3 and 10 and put them on Wikicommons, and that's where I got my picture here!
I later discovered that are also 'Lorentzian Dynkin diagrams' which are almost as good as the hyperbolic ones, whose dimension can exceed 10. For example, there's one in 26 dimensions that's connected to bosonic string theory, and I described it using octonions here:
In 1970, Belinksii, Khalatnikov and Lifschitz discovered that when you run time backwards toward the Big Bang, a homogeneous universe behaves like a billiard ball. As you run time back, the universe shrinks, but also its shape changes. Its shape moves around in some region of allowed shapes... and it 'bounces' off the 'walls' of this region!
These guys considered the simplest case: a universe with 3 dimensions of space and 1 dimension of time, containing gravity but nothing else. In this case the region of allowed shapes is a triangle in the hyperbolic plane. I showed it to you in my last diary entry.
So, running time backwards in this kind of universe is mathematically very much like watching a frictionless billiard ball bounce around on a strangely curved triangular pool table.
But you can play the same game for other theories: gravity together with various kinds of matter, in universes with various numbers of dimensions. And when people did this, they discovered something really cool. Different possibilities gave different kinds of pool tables!
When space has some number of dimensions, the pool table has dimension one less. As far as I know, it's always sitting inside 'hyperbolic space', a generalization of the hyperbolic plane. And it's always a piece of a hyperbolic honeycomb — a very symmetrical way of chopping hyperbolic space into pieces.
The picture here, drawn by Roice Nelson, shows a hyperbolic honeycomb in 3-dimensional hyperbolic space. So, one tetrahedron in this honeycomb could be the 'pool table' for a theory of gravity where space has 4 dimensions. (In fact it doesn't quite work like this: we have to subdivide each tetrahedron shown here into 24 smaller tetrahedra to get the 'pool tables'. But never mind.)
Even better, these stunningly symmetrical patterns arise from what I called spacetime crystals. The technical term is 'hyperbolic Dynkin diagrams', and I told you about them earlier. The picture here, in 3 dimensions, arises from a spacetime crystal in 4 dimensions. That's how it always works: the crystal has one more dimension than the pool table.
And here's the really amazing thing: mathematicians have proved that the highest possible dimension for a spacetime crystal is 10. This gives you a 9-dimensional pool table, which is the sort of thing that could show up in a theory of gravity where space has 10 dimensions.
And there is a theory of gravity in where space has 10 dimensions: it's called 11-dimensional supergravity, because there's also 1 dimension of time in this theory. String theorists like this theory of gravity a lot, because it seems to connect all the other stuff they're interested in.
It turns out this particular theory of gravity gives a spacetime crystal called E10. There are several other 10-dimensional spacetime crystals, but this is the best.
For a while I've been thinking that we should be able to describe E10 using the octonions, an 8-dimensional number system that shows up a lot in string theory. I had a guess about how this should work. And last week, my friend the science fiction writer Greg Egan proved this guess is right!
For the details, go here:
This result probably came as no surprise to the real experts on cosmological billiards — I'm no expert, I just play a game now and then. Here is a nice introduction by a real expert:
And here are some more detailed papers:
November 4, 2014
Looping Lorentzian lattice!

A rapidly moving observer will see time (the vertical axis) and space (the horizontal axis) in a different way than you do at rest. As their speed increases the warping increases.
Each black dot is a point in spacetime. As viewed by faster and faster observers, it moves along a hyperbola. But after a while, the whole lattice of black dots gets back to the same pattern it started with!
The warpings of spacetime shown here are called Lorentz transformations. Greg Egan made this movie to illustrate how we can do a Lorentz transformation to a lattice in spacetime and get back the same lattice. This is the one of the symmetries that you get in what I was calling a 'spacetime crystal' — technically, a lattice coming from a symmetrizable hyperbolic Dynkin diagram.
For many beautiful pictures related to looping Lorentzian lattices, try:
The set of all Lorentzian lattices where each parallelogram has area 1 forms a 3d space with a trefoil knot removed! As we keep applying Lorentz transforms to a lattice, it traces out a curve in this space.
Here is a looping Lorentzian lattice in 3d spacetime, again made by Egan:
.gif)
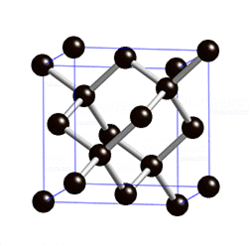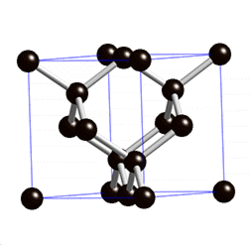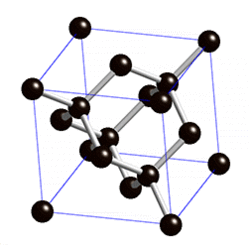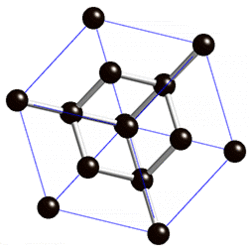
Diamonds are one of hardest known substances. They're made of carbon, with each atom connected to 4 others in a pattern called the diamond cubic.
The same pattern appears in crystals of silicon, germanium, and tin. These are other elements in the same column of the periodic table. They all like to hook up with 4 neighbors.
The diamond cubic is elegant but a bit tricky. Look at it carefully here! We start by putting an atom at each corner of a cube. Then we put an atom in the middle of each face of the cube. So far, this is called a face-centered cubic.
But then: the tricky part! We put 4 more atoms inside the cube. Each of these has 4 nearest neighbors, which form the corners of a tetrahedron.
What are the coordinates of these points? It's good to start with a 4×4×4 cube. Its corners are:
$$ (0,0,0) \; (4,0,0) $$ $$ (0,4,0) \; (4,4,0) $$
$$ (0,0,4) \; (4,0,4) $$ $$ (0,4,4) \; (4,4,4) $$
The middles of its faces are
$$ (2,2,0) \; (2,0,2) \; (0,2,2) $$ $$ (2,2,4) \; (2,4,2) \; (4,2,2) $$
We can take the four extra points to be $$ (1,1,3) \; (1,3,1) \; (3,1,1) $$ $$ (3,3,3) $$
So, here's a nice way to describe all the points in the diamond cubic. They're points \((x,y,z)\) where:
Tricky, eh?
Part of why it's tricky is that there was a choice. We could also switch the 1's and 3's in the four extra points, using $$ (1,1,1) $$ $$ (3,3,1) \; (3,1,3) \; (1,3,3) $$
instead. Then we'd get a diamond cubic with points \((x,y,z)\) where:
Puzzle 1: Is the diamond cubic a 'lattice' in the mathematical sense? A lattice is a discrete set of points that is closed under addition and subtraction.
Puzzle 2: take n-tuples of numbers where:
Does this give you a lattice? The answer may depend on n.
Puzzle 3: For experts: when you get a lattice in Puzzle 2, what is this lattice called?
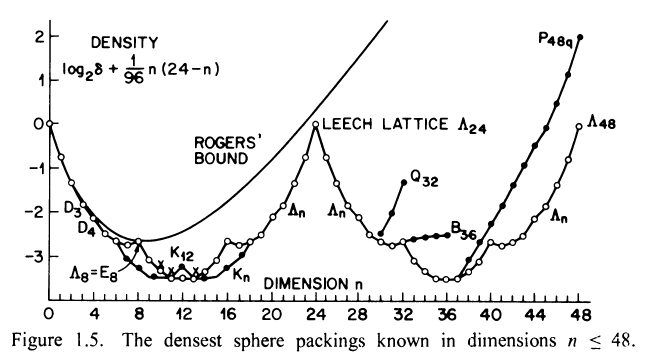
My main hobby these days is working with Greg Egan on lattices. Roughly, these are repeating patterns of points, like the centers of atoms in a crystal. But you can study lattices in different dimensions — and a lot of fun happens in 24 dimensions!
If you look for the densest ways to pack spheres in different dimensions, you'll be led to some interesting lattices. In 3 dimensions, the usual way of stacking oranges gives the 'D3 lattice': when you center your spheres at points of this lattice, each sphere touches 12 others. This is known to be the densest packing of spheres in 3 dimensions. It's also called the 'face-centered cubic', and I discussed it in my September 16th diary entry, as well as yesterday's.
In 4 dimensions the densest known sphere packing comes from the D4 lattice, where each sphere touches 24 others.
These D lattices are easy to build: you draw a higher-dimensional checkerboard with alternating red and black hypercubes, and put a dot in the middle of each red hypercube.
When you pack sphere using these D lattices in higher and higher dimensions, there's more and more room left over between your spheres. And when you get to 8 dimensions, something funny happens! There's so much room left that you can slip in another whole set of spheres packed the same way!
So, you can double the density with this improved lattice. It's called the 'E8 lattice', and you see it as a peak in the graph here. With this lattice, each sphere touches 240 others. Nobody has proved that this is the densest sphere packing possible in 8 dimensions. But in 2009, Henry Cohn and Abhinav Kumar proved that no other packing can beat its density by a factor of more than
So, I'm willing to bet that it's the best.
What I really like about 8 dimensions is that there's an 8-dimensional number system where you can add, subtract, multiply and divide.
I'm sure you know how a 1-dimensional ruler is labelled by ordinary real numbers. You can add, subtract, multiply and divide those. If you try to do this trick in higher dimensions, you'll notice something weird: you can only do it in dimensions 1, 2, 4, and 8.
In 2 dimensions you can use the complex numbers, and in 4 you can use the quaternions. In 8 dimensions you can use the octonions, and that's where the game ends! So the octonions are special. They play a role in string theory — so if string theory ever turns out to be right, maybe the octonions will actually count as useful. Right now they're just amazingly beautiful and lots of fun.
But back to lattices! The simplest lattice lives in 1 dimension: it's the evenly spaced numbers on your ruler, called integers:
You can add, subtract and multiply integers and get integers... but not divide them: that takes you out of the integers.
There are versions of the integers for complex numbers, quaternions and octonions too! The Hurwitz integral quaternions form the D4 lattice that I mentioned earlier. And the Cayley integral octonions form the E8 lattice. It's actually the arithmetic of these integral octonions that fascinates me, more than the sphere packing business.
But as you can see from the graph, there's a really interesting mountain peak called the 'Leech lattice'. This gives the densest known way to pack spheres in 24 dimensions. Nobody has proved it's the best — but Cohn and Kumar proved that no other packing in this dimension can beat its density by more than a factor of
It's a lot harder to describe the Leech lattice than the others I mentioned so far. Each sphere touches 196,560 others... and the pattern is rather tricky.
But 24 is 3 times 8, so you might hope to build the Leech lattice from 3 copies of the E8 lattice... and you can! But you need a fairly clever trick. Various people have described this trick in different ways, but I like Greg Egan's the best. I explain this here: this series:
It relies on a great feature of the E8 lattice. You can rotate it in a way that turns every point by the same specific angle, and expand it by factor of \(\sqrt{2}\), and this transformation maps the E8 lattice into itself. Any way of doing this gives a way to build the Leech lattice.
The graph of densities here is taken from Conway and Sloane's book Sphere Packings, Lattices and Groups. They take spheres of radius 1, work out the density of sphere centers, take its logarithm in base 2... and then add \(n(24-n)/96\). This is a parabola peaked at 12. I find this last touch a bit distracting.
Here's the paper I mentioned:
For more, see the comments on my G+ thread.

Man was winged hopefully. He had in him to go further than this short flight, now ending. He proposed even that he should become the Flower of All Things, and that he should learn to be the All-Knowing, the All-Admiring. Instead, he is to be destroyed. He is only a fledgling caught in a bush-fire. He is very small, very simple, very little capable of insight. His knowledge of the great orb of things is but a fledgling's knowledge. His admiration is a nestling's admiration for the things kindly to his own small nature. He delights only in food and the food-announcing call. The music of the spheres passes over him, through him, and is not heard.Yet it has used him. And now it uses his destruction. Great, and terrible, and very beautiful is the Whole; and for man the best is that the Whole should use him.
But does it really use him? Is the beauty of the Whole really enhanced by our agony? And is the Whole really beautiful? And what is beauty? Throughout all his existence man has been striving to hear the music of the spheres, and has seemed to himself once and again to catch some phrase of it, or even a hint of the whole form of it. Yet he can never be sure that he has truly heard it, nor even that there is any such perfect music at all to be heard. Inevitably so, for if it exists, it is not for him in his littleness.
But one thing is certain. Man himself, at the very least, is music, a brave theme that makes music also of its vast accompaniment, its matrix of storms and stars. Man himself in his degree is eternally a beauty in the eternal form of things. It is very good to have been man. And so we may go forward together with laughter in our hearts, and peace, thankful for the past, and for our own courage. For we shall make after all a fair conclusion to this brief music that is man.
This is the end of Olaf Stapledon's Last and First Men. An early SF classic, it describes the history of humanity for the next two billion years, embodied 18 different species and living on several planets. It was written in 1930, so try to forgive the sexist language and various kinds of naivete. You can read the whole book here:
Craig Kaplan has been taking ideas from Islamic wall tilings and adapting them to spheres. It's a great way to bring new life to an glorious old tradition.
See that star with 10 points and 5 nearest neighbors? That's 5-fold symmetry. You can't get perfect 5-fold symmetry in a tiling of the plane. The best you can do is fake it in various ways — and by 1200 AD the great tile masters of Afghanistan, Iran, Morocco and Turkey had figured out most of these ways.
Patterns with decagons and pentagons that fool the eye into thinking there's 5-fold symmetry! Quasiperiodic tilings — later rediscovered by Penrose — that never quite repeat but have 5-fold symmetry on average. Their discoveries were remarkable.
But when you tile a sphere, the dodecahedron comes to your aid: it has 5-fold symmetry, and things the old tile experts did with hexagons, you can now do with pentagons! It's like a whole new world.
And the world expands even more when you use the hyperbolic plane: then you can get 7-fold symmetry, 8-fold symmetry and so on. Kaplan has also studied that.
If you look carefully at this pattern, you'll see every star with 10 points is surrounded by 5 stars with 9 points... and every star with 9 points is surrounded by 6 stars, which alternate between having 9 and 10 points. The stars with 10 points are the centers of the faces of a dodecahedron, so there are 12 of them. The stars with 9 points are at the vertices of this dodecahedron, so there are 20 of them.
The whole pattern is made of little things that look almost like triangles, but have bent edges.
Puzzle: how many of these little things are there?
I thank Layra Idarani for making me pay attention to these details.
This image was created by TaffGoch based on a design by Craig Kaplan. For more beautiful stuff, check out this page:
TaffGoch has a lot of great stuff on DeviantArt.

Europeans have trouble understanding the USA's love affair with guns. I have trouble too. But here is one of our folk heroes: Annie Oakley.
Born in 1860 in a log cabin in Ohio, at the age of 10 she was 'bound out' to a nearby family to help care for son, on the false promise of fifty cents a week and an education. Being 'bound out' was pretty common for poor children: at best it was like becoming an apprentice, at worst it was pretty much like slavery. Annie always called this family "the wolves" — and at the age of 12 she ran away back to her mother. She'd started hunting at the age of 8 to help support her brothers and sisters, and she got good at shooting. Really good.
A travelling show called Baughman & Butler came to town. Butler was a marksman. He placed a $100 bet that he could beat any local shooter. The last thing he expected was a five-foot-tall, 15-year old challenger named Annie! They took turns. After missing on his 25th shot, Butler lost the match.
A year later, he married Annie.
In 1885, they joined Buffalo Bill Cody's travelling circus. Buffalo Bill is another one of America's famous shooters. He began his career by exterminating buffaloes and Indians. But by this time, he was running a show called 'Buffalo Bill's Wild West'. It featured notables such as Wild Bill Hickock and Sitting Bull — a chief of the Lakota tribe who had helped defeat Custer in a famous battle.
By this point, Annie Oakley had become almost superhuman. Her most famous trick was to split a playing card, edge-on, and put several more holes in it before it could touch the ground — all using a rifle at 90 feet.
According to the Encyclopedia Brittanica:
Oakley never failed to delight her audiences, and her feats of marksmanship were truly incredible. At 30 paces she could split a playing card held edge-on, she hit dimes tossed into the air, she shot cigarettes from her husband's lips, and, a playing card being thrown into the air, she riddled it before it touched the ground.
One day Chief Sitting Bull was watching when
Oakley playfully skipped on stage, lifted her rifle, and aimed the barrel at a burning candle. In one shot, she snuffed out the flame with a whizzing bullet.
Later the show went to Europe. At his request, she used a bullet to knock the ashes off a cigarette held by Kaiser Wilhelm II. Later some people later said that if Annie had shot the Kaiser, she could have prevented World War I.
In fact, after the war started, Oakley sent a letter to the Kaiser requesting a second shot. The Kaiser did not reply.
And so on. Teddy Roosevelt turned down her request to lead a company of woman sharpshooters in the Spanish-American war. She won 54 of 55 libel suits against various newspapers when the publisher Randolph Hearst spread a false story that she'd been arrested for stealing to support a cocaine habit. At the age of 62, she won a contest by hitting 100 clay targets in a row from a distance of 16 yards.
At the age of 66, she died of pernicious anemia. Her husband Butler was so grieved he stopped eating and died a couple of weeks later.
Apart from Hearst, Annie Oakland and her husband are the only Americans named in this story who never killed anyone with a gun. Chief Sitting Bull was later shot and killed by the police.
I got interested in Annie Oakley last night while watching an episode of TV's best-kept secret, The Murdoch Mysteries. You can see it on Netflix. In this episode, Buffalo Bill's travelling show comes to Toronto, someone gets shot... and Annie Oakley is one of the suspects. I was wondering how accurate it all was. It seems pretty realistic, though I don't know if Buffalo Bill's Wild West ever went to Toronto.
I got my information here:
In Alabama, 1/3 of black men cannot vote.
Why not? Because if you go to prison in that state, you may never be allowed to vote after that. Since black men are imprisoned at an extremely high rate, many can't vote.
There are 11 states in the US with laws like this. Others deny the vote for shorter periods of time — for example, while you're in prison or are on parole. Here are some of the consequences:
These statistics are old, so they will have changed. But I don't think they've gotten better — except that in the last 20 years, three states stopped doing this.
An estimated 5.3 million Americans are denied the right to vote based on their felony convictions, 4 million of whom are out of prison. About a third of them are black, including 13% of all African-American men.
For up-to-date information, go to the Sentencing Project website:
They list percentages of African American citizens who are denied the right to vote, not African American men, so the figures look slightly less shocking. More details are in this report:
but for African American men the most up-to-date comprehensive statistics I've found are from 1996, so that's what I used in my list.
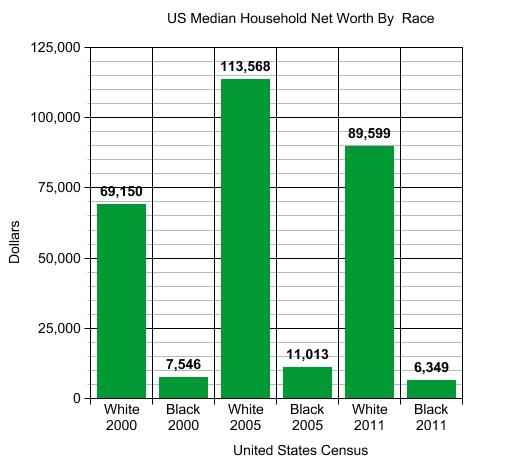
In 2005, white US households were about 10 times more wealthy than black ones, as measured by median net worth. By 2011, they were 14 times as wealthy!
From 2005 to 2011, the Great Recession knocked American white households' median net worth down by 21%. But for blacks, it dropped by 42%.
The numbers are from the US Census.

This is the 'Golay code'. Each row in this picture shows a string of 24 bits. There are 12 rows. If you look at any two rows, you'll see they differ in at least 8 places.
Here's how to get the Golay code. Take a 12 × 12 square of bits with all 0's except for 1's down the diagonal — you can see that at left here. Take another 12 × 12 square of bits that tells you when two faces of a dodecahedron share an edge: 0 if they do, 1 if they don't. Stick these squares together and you get the Golay code!
Some guys around here keep asking if the math I talk about is good for anything. In this case it is!
The Voyager 1 and 2 spacecraft needed to transmit hundreds of color pictures of Jupiter and Saturn in their 1979, 1980, and 1981 fly-bys. They had very little bandwidth, so they needed a good error-correcting code. They used the Golay code!
The point is that we can use the rows of this picture as code words. If we take some rows and add them — adding each entry separately, mod 2 — we get more code words. We get a total of 212 = 4096 code words. Strictly speaking, its this set of code words that is the Golay code.
These code words have a cool property: it takes at least 8 errors to turn any code word into any other. So, we say the Hamming distance between any two code words is at least 8. In fact, the Golay code is the only code with 24-bit code words where the Hamming distance between any two is at least 8.
There's a whole theory of codes like this, and this is an especially good one. You can transmit 12 bits of data with 24 bits... but since the Hamming distance between code words is big, someone can understand what you meant even if there are lots of errors! So, the Golay code is useful for transmitting data in a noisy environment.
But the reason I like the Golay code is that it has a big and important symmetry group. Its symmetry group is called M24 - one of the amazing things called Mathieu groups. It has
elements. It's connected to many other symmetrical things in math: for example, it acts as symmetries of the Leech lattice, the densest way to pack balls in 24 dimensions.
To be more precise, this code here is called the extended binary Golay code. You can learn more about it here:
Puzzle: I said the symmetry group of this code is M24. But what do I mean, exactly, by a 'symmetry' of this code?
The extended binary Golay code is not only good for outer space. In 1993, the US government issued standards for high frequency radio systems. They require using this code for "forwards error correction" in "automatic link establishment"! See page 51 here:
November 22, 2014
The virus has landed!
This is a virus called a T4 bacteriophage. It has landed on a bacterium. Now it's getting ready to lower its tail, puncture the bacterium's cell wall, and inject its DNA.
When this happens:
This deadly machine is only 0.2 micrometers tall. Its DNA — the instruction book that makes everything work — is contained in the head, which is shaped like an icosahedron. The DNA is 169,000 base pairs long, and it codes for 289 proteins. Biologists understand it quite well now.
This picture is not a photograph; it was made by Mike Smith for a company called Xvivo Scientific Animation.
This is boron carbide, an extremely hard ceramic material used in macho gear like tank armor, bulletproof vests, and engine sabotage powder.
(Engine sabotage powder? Yes, you can pour this into the oil supply, and it will make a car engine grind itself to death.)
If diamond has a hardness of 10, this comes in at 9.497. But its crystal structure is even cooler than diamond!
A group of 12 boron atoms likes to form an icosahedron. You can see 8 of these icosahedra here — the green things. These form the corners of a rhombohedron — a kind of squashed cube. These repeat over and over, forming a rhombohedral lattice.
But that's not all! The icosahedra are connected by struts! These struts have carbon atoms at their ends and a boron in the middle. Only one strut is shown in detail here. The carbon atoms are the black balls and the boron is the little green ball.
Overall there are 4 boron atoms per carbon atom, so people call boron carbide B4C.
Puzzle 1: Why are there 4 borons per carbon? I haven't done the counting, so I don't understand this.
Puzzle 2: What's the difference between a rhombus and a parallelogram?
Puzzle 3: What's the difference between a rhombohedron and a paralleliped?
Puzzle 4: What's the difference between a rhombohedral crystal and an 'orthorhombic' crystal?
Another macho application of boron carbide is to shielding and control rods for nuclear reactors! The reason is that boron can absorb neutrons without forming long-lived radioactive isotopes.
The structure of boron carbide has even more subtle features, which I don't understand. Maybe I'm not looking at the pictures carefully enough!
Puzzle 5: Where are the octahedra made of boron atoms? For clues, read this:
The picture here was made by 'Materialscientist' and placed on Wikicommons.
For answers to some of the puzzles, see the comments to my G+ post. For another marvelous boron molecule, check out my July 21st diary entry on borospherene.