
Gauge theory describes the parallel transport of point particles using the formalism of connections on bundles. In both string theory and loop quantum gravity, point particles are replaced by 1-dimensional extended objects: paths or loops in space. This suggests that we seek some kind of "higher gauge theory" that describes the parallel transport as we move a path along a path of paths. To find the right mathematical formalism for this, it seems we must "categorify" concepts from differential geometry, replacing smooth manifolds by smooth categories, Lie groups by Lie 2-groups, Lie algebras by Lie 2-algebras, bundles by 2-bundles, sheaves by stacks or gerbes, and so on. In this talk we provide an overview of work so far on this topic, with an emphasis on open problems and some tantalizing relationships between Lie 2-groups and central extensions of loop groups.
I will describe aspects of this joint work on the construction of a 2-group associated to the Kac-Moody central extension of the loop group. I will explain how this construction can be understood in terms of "categorified bundles", i.e. gerbes and 2-bundles. Finally I will show how the 2-group we construct is related to the group String(n) which plays an important role in the work of Stolz and Teichner on elliptic objects.
A major result in differential geometry is Ado's theorem, which states that every finite-dimensional Lie algebra comes from some Lie group. We seek to prove a higher-dimensional analog of this statement for Lie 2-groups and Lie 2-algebras, which are categorified versions of Lie groups and Lie algebras where we have replaced the associative law and Jacobi identity, respectively, by natural isomorphisms called the "associator" and the "Jacobiator". While this problem remains open, we describe progress toward a solution by examining the Lie 2-algebras gk, each having the simple Lie algebra g as its Lie algebra of objects, but with a Jacobiator proportional to a real number k and built from the Killing form. It seems that except for k=0, there does not exist a Lie 2-group whose Lie 2-algebra is isomorphic to gk. However, we can construct for integral values of k an infinite-dimensional Lie 2-group whose Lie 2-algebra is equivalent to gk. Moreover, these Lie 2-groups are closely related to the Kac-Moody central extensions of loop groups!
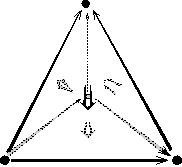
Ordinary gauge theory involves a groupoid in which the objects
are points of spacetime and the morphisms are paths. Higher gauge theory
goes further and uses a 2-groupoid where the objects are points, the
morphisms are paths, and the 2-morphisms are "paths of paths" -
which we use to describe surfaces traced out by the motion of
strings. So, higher gauge theory is naturally tied to categorification.
Here we show how categorifying the concepts of bundle
and connection yields the concepts of "2-bundle"
and "2-connection".
Just as a connection can be locally described
as a Lie-algebra-valued 1-form, a 2-connection
is locally described by a Lie-algebra-valued 1-form together with a
Lie-algebra-valued 2-form. A 2-connection gives well-behaved parallel
transport along paths and surfaces if a quantity called its
"fake curvature" vanishes.
In this talk we sketch these ideas and discuss how
2-connections on 2-bundles are related to connections on
nonabelian gerbes.
Higher Gauge Theory (II)
John Baez
Gauge theory describes how
point particles transform as they trace out paths
in spacetime using the formalism of connections on bundles.
In higher gauge theory, we describe the parallel transport
of strings as they sweep out surfaces in spacetime
using "2-connections" on "2-bundles".
Our talks are based on these papers:
For some other work on this topic, see:
© 2005 John Baez
baez@math.removethis.ucr.andthis.edu
home